Category: Mathematics Questions

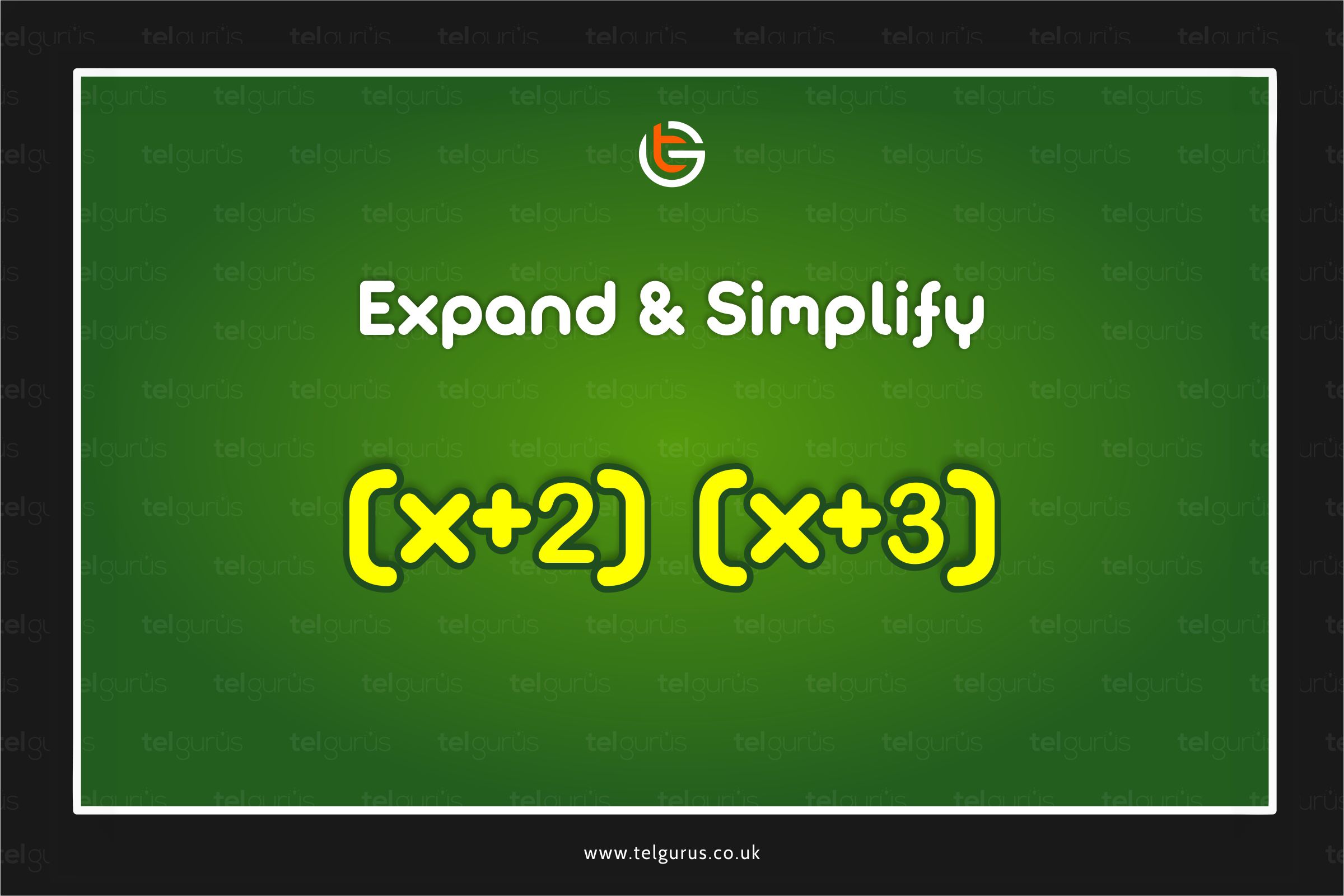
Calculation of integrals is known as integration. Integration is an inevitable part of calculus in mathematics akin to differentiation.   

Integration is a really vast topic. Integration is a method of adding up parts of a function to get the whole entity.  

It’s more like a whole pizza and the pieces of it are the differentiable functions which can be integrated to get the whole pizza back.   

Integration can be used to solve the following types of problems:  

• Simplifying a problem function, when its derivatives are given.  
• It helps to find the area bounded by a graph of a function given under certain conditions.   

The symbol used for integration is   ∫. 

For example: 

We know that cosine x is the derivative of sine x. This means sine x or sin x is the antiderivative or integral of cosine x (cos x). 

Integration is exactly the opposite of differentiation.  

While differentiation means segregating into small pieces, integration is the summing of those small pieces to get the thing back.  

Calculation of small addition problems is an easy task that can be done manually or by using calculators as well. But, for high-end additions where the limits could reach infinity, we use integration methods.   

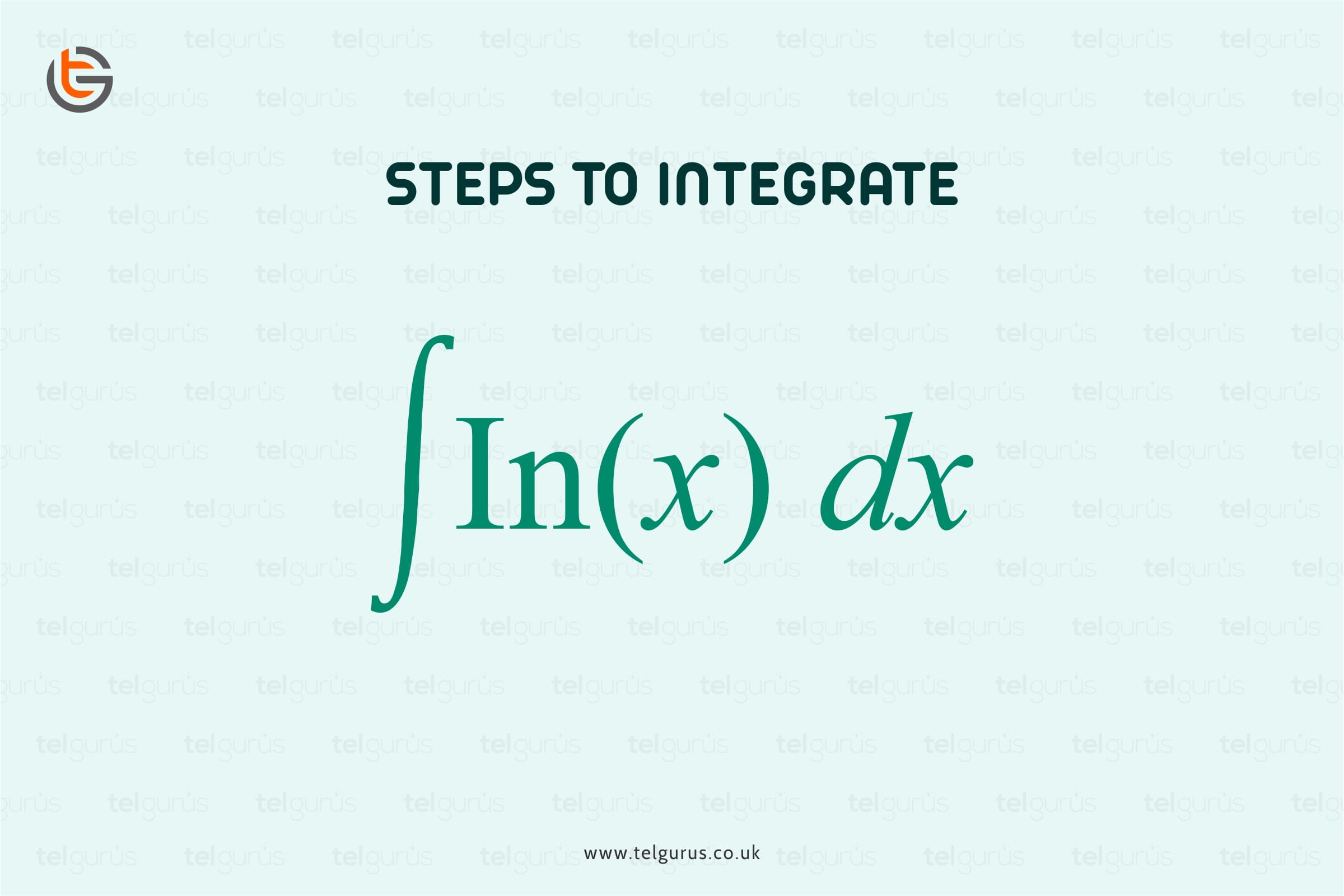
Now, the question is how to integrate ln ( x )? 

In this question we will use the method of Integration by Parts. 

 Integration by parts is a method of integration that is used when two functions are represented in multiplication form. 

According to Integration by parts: 

First function * (integral of second function) – integral of (derivative of first function * (integral of second function))                                   

Some Important Points:  

• When both the functions in the product can be integrated separately and one of them is of the form x n, take this as the first function. 
•  If one of the two functions in the product is a logarithmic or an inverse trigonometric function, it must be taken as the first function. 
• The rule of parts can also be applied to single functions and is especially useful in the case of logarithmic or inverse trigonometric functions. For this, unity i.e. 1 become the second function.  

By looking at the above points, we can conclude that: 

∫ f (x) g’ (x) dx = f(x) g(x) –  ∫ f’(x) g(x). dx  

But, in ln( x ) there is only one function and integration by parts is applied only when there are two functions. 

So, here we can take another function as 1. 

Therefore take ln ( x )  as the first function  and  1 as the second function. 

f(x) = ln (x) 

f’(x) = 1/x 

g(x) = x 

g’(x) = 1 

By applying the integration by parts rule: 

∫ ln ( x )  . 1 dx  =  ln ( x )   ∫ 1 dx  –  ∫  d / dx  (  ln ( x )   )  ∫ 1 . dx  

                             = ln ( x ) .  x   –   ∫  (  1 / x  )  . x  . dx 

                             = x  ln ( x )   –   ∫  1 . dx  

                             =  x  ln ( x )  –  x  +  c  

So , The integral of  ln ( x )  is    x  ln ( x )  –  x  +  c  

For every integration, we add a constant c to make sure that nothing is lost.

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

What is discriminant in math?

Discriminant in math is a function of the coefficients of the polynomial. Discriminant Symbol  

The symbol of discriminant is   “D“. 

To find the number of real roots of quadratic equation first we should know what is the quadratic equation?   

A quadratic equation is that in which the maximum power of variable is 2  

General Form of Quadratic Equation

A quadratic equation is in the form: \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~

The discriminant of a quadratic equation \displaystyle ~a{{x}^{2}}~+~bx~+~c~ is in terms of a , b , and c is  

\displaystyle D~=~{{b}^{2}}-~~4ac

Let us clear the concept of discriminant with help of some examples: 

Example1 

Find the discriminant of  \displaystyle ~2{{x}^{2}}~+~3x~+~3~=~0~

Sol:  Compare the given expression with \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~

a=2

b=3

c=3

The discriminant of the given equation is 

By applying the formula \displaystyle D~=~{{b}^{2}}-~~4ac

                      =  \displaystyle ~{{3}^{2}}~-~4(2)(3)~

                      = 9 – 24

                      = − 15 

Thus, the discriminant of the given equation is  − 15

There are three cases for the discriminant: 

Case 1: 

\displaystyle ~{{b}^{2}}~-~4ac~>~0~

If the discriminant is greater than zero, this means that the quadratic equation has two real, distinct roots. 

Let’s understand that with an example: 

\displaystyle ~{{x}^{2}}~-~5x~+~2~=~0~

Here, a = 1   , b=  – 5 ,  c= 2 

Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac

                     =  \displaystyle ~{{(-5)}^{2}}~-~4(1)(2)~

                      = 17 

Discriminant  is greater than zero i.e. 17 > 0. 

Therefore, the roots of the above equation will be real and distinct.  

Case 2: 

When, \displaystyle ~{{b}^{2}}~-~4ac~<~0~

If the discriminant is less than zero then the quadratic equation has no real roots.  

Example: 

\displaystyle ~3{{x}^{2}}~-~2x~+~1~=~0~

Here,  a= 3  ,   b = 2  , c= 1  

Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac

                              = \displaystyle ~{{(2)}^{2}}~-~4(3)(1)~

                              = 4  −  12 

                               =  − 8 which is < 0 

In above equation value of the discriminant is less than zero. Therefore, the roots of this quadratic equation are not real.  

Case 3: 

 When, D or \displaystyle ~{{b}^{2}}~-~4ac~=~0~

If the discriminant   is equal to zero, this means that the quadratic equation has two   real, identical roots .  

Example : 

\displaystyle ~{{x}^{2}}~+~2x~+~1~=~0~

Comparing the above equation with general quadratic equation  \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~

We get a = 1, b  =  2,  c  =  1

Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac

                               = \displaystyle ~{{(2)}^{2}}~-~4(1)(1)~

                               = 4 − 4

                               = 0

In the above equation discriminant is zero therefore the above equation has two real, identical roots.  

So, from above discussion we can conclude that  

• • If discriminant <  0,  there are  2  imaginary roots . Imaginary roots are those which are in the form of i which is known as ita in mathematics as discriminant is negative.  

• • If discriminant > 0, there are 2 real, distinct roots.  
  • If discriminant = 0, there is one real root and the value of that root is   \frac{{-b}}{{2a}}

So, the discriminant of a quadratic polynomial provides information about the properties of the roots of the polynomial. 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

Let’s first understand what are prime numbers and factors. 

What is a factor? 

A factor is a number that divides the given number evenly or completely. This means on dividing, the remainder will be zero.  

For example, 3 and 2 are factors of 36 i.e. they divide 36 completely.  

Also on dividing 36 by 2 or 3, the remainder turns out to be zero.  

What are prime numbers?  

Prime numbers are those numbers that have only two factors i.e. 1 and the number itself.  

No other number is able to divide them completely.  

For example, 3 is a prime number and just has two factors 1 and 3. 

The numbers that are not prime are called composite numbers.  

Book Your 60-minutes Free Trial class NOW!

36 and its factors  

The number 36 is an even number i.e. it has 6 at its unit’s place. Every even number is composite.  

So, let’s begin finding factors of 36. 

Method 1: Division Method  

We start by 2: 

1. 36 on dividing by 2 gives 16 

This means we can represent 36 as a multiplication of 18 and 2.  

36 = 18 * 2 ……………………………………………………. Equation (1) 

2. Let’s further break it. Here, 18 is again an even number s it ends with 8 at the unit’s place and 2 is a prime number as it has just two factors 1 and 2. 

So, 18 can further be expressed into a product of two numbers i.e.  

18 = 9 * 2 

Replacing the value of 18 in equation (1), we get 

36 = 9 * 2 * 2 ……………………………………………………. Equation (2) 

3. Now we have got two 2’s which are prime and a 9 which we know is a composite number.  

9 is obtained by multiplying two 3’s i.e. 9 can be expressed as a product of two 3’s. 

9 = 3 * 3; 

We can replace 9 in equation (2) and we get: 

36 = 3 * 3 * 2 * 2 

 Now, all the numbers or factors of 36 are prime. No further breaking is required. 

So, the 36 can be written as a product of two 3’s and 2’s or; 

36 = 2^2 * 3^2 

Book Your 60-minutes Free Trial class NOW!

Another way to find factors is the prime factorization method or upside division method.

Method 2: Prime factorization  

Method:  

• With the help of divisibility rules, first, you need to find the smallest factor of the given number. Divide and write the quotient.   
• The process is repeated with every quotient obtained till the quotient comes out to be 1. 

Let’s do the prime factorization of 36: 

2	36
2	18
3	9
3	3
	1

Prime factorization is the best way to obtain a number as a product of all prime numbers.  

So, by looking at the left side we can easily note that 

36 = 2 * 2 * 3 * 3 

Method 3: Factor tree method 

The factor tree method is one of the most effective in grasping the concept of expressing a number into a product of prime numbers as it involves visualization.  

Here we take the number in the node and the child nodes contain the numbers or factors of that number. 

For that, we just need one relation or any two numbers that on multiplication give the desired number.  

So, for 36 we can either go for 2 * 18 or we can also pick 6 * 6 or 9 * 4 

For convenience we take 36 = 2 * 18. 

Now, multiply all the last child nodes. This will give you the answer. 

36 = 2 * 2 * 3 * 3 or 2^2 * 3^2 

Book Your 60-minutes Free Trial class NOW!

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

Algebra is one of the pillars of mathematics that needs a sound understanding and mastering of concepts to ace it.  

Simplification of expressions is one of the most commonly asked questions from algebra that help you change complex terms into simpler ones or compact forms.  

What is an algebraic expression?

It’s a mathematical term that is a mixed bag of variables and constants joined together with mathematical operators such as +, – , / and *. 

So, Let’s see the various methods to solve (x + 3) (x +5). 

While solving any mathematical expression, always follow the concept of BODMAS which states the order of mathematical operations. 

Always start with opening brackets followed by solving division, multiplication, addition and then at last subtraction. 

Book Your 60-minutes Free Trial class NOW!

There are mainly three methods of solving any algebraic expression involving brackets. 

1. Using FOIL Method  
2. Using Distributive property 
3. Direct formula 

So, let’s discuss them one by one in detail. 

1. Using FOIL Approach 

FOIL approach is basically used by most of the students to simplify the algebraic expression. It’s an acronym that helps you remember the sequence of multiplication. 

After multiplication, just sort the like terms and then just add.  

What does FOIL stand for? 

F: Multiply the first two terms of the expression  

O: Multiple outer two terms of each bracket. 

I: Now, multiple inside two terms.  

L: multiply the last two terms from the bracket 

Let’s apply this FOIL approach to our question (x + 3) (x + 5) 

As per F, lets multiply first term from both brackets i.e. x in both cases. This comes out to be \displaystyle x.x={{x}^{2}}. 

Moving on to O, we multiply the outer two terms i.e. x and 5 which comes out to be 5x. 

I mean multiplying inner two terms i.e. 3 and x. On multiplying this becomes 3x. 

Last, as per “L”, we multiply 3 and 5, the last two terms that give you 5 * 3 = 15. 

So, we get the four terms as x^2, 5x, 3x and 15. 

Let’s separate the like terms and add all these terms. 

\displaystyle {{x}^{2}}+(5x+3x)+15

= \displaystyle {{x}^{2}}+8x+15 

So, on simplifying (x + 3)(x + 5) we get   \displaystyle {{x}^{2}}+8x+15 

2. Using Distributive property 

You can simplify the algebraic expressions by using the distributive property. 

How it works:  

(x + a) (x + b) = x (x + b) +a (x+ b) 

By using the above property, we multiply the second bracket with every element of first bracket. 

(x + 3)(x + 5) 

= x (x + 5) + 3 (x + 5)   

= x.x + x.5 + 3.x + 3*5  (on opening the brackets) 

= \displaystyle {{x}^{2}}+5x+3x+15 

So, by combining the like terms we get our final answer 

=  \displaystyle {{x}^{2}}+8x+15 

Book Your 60-minutes Free Trial class NOW!

3. Direct formula 

The above two methods are best when you are going for subjective papers. 

 When you are aiming at competitive exams where every second matters, you just can’t afford to go for a long method. 

We have a formula, you just need to substitute the values and that’s it. 

You get the answer within a blink of an eye. 

For solving (x + a) (x + b), use the formula x^2 + (a + b)x + ab 

As per our question, the values of a and b are 3 and 5 respectively. 

So, substituting them in the formula we get 

=  \displaystyle {{x}^{2}}+(3+5)x+3*5 

= \displaystyle {{x}^{2}}+8x+15 

The third was one was pretty easy and can be used to simplify the problem very quickly and efficiently. 

 

Book Your 60-minutes Free Trial class NOW!

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

To solve (x + 2) (x + 3), there are mainly two methods. Both the methods are very simple.

While multiplying algebraic terms you need to be very careful about the signs. Multiplying two unlike signs will result in a negative term while multiplying two similar signs will always yield a positive term.

Solve a few of them using these methods and you will easily master these.

The two methods are:

1. Using distributive property
2. Using FOIL method

Let’s discuss them one by one:

Solving (x+2) (x+3) using distributive Property.

According to distributive properly, we have to first distribute the second term w.r.t. the first term.

So, let’s distribute:

x (x + 3) + 2 (x + 2)

Now, open the brackets one by one by multiplying

= x.x + 3.x + 2 (x + 2)

= x² + 3x + 2.x + 2.2

= x² + 3x + 2x + 4

Now, combining the Like terms we get:

= x² + (3x + 2x) + 4

= x² + 5x + 4

So, we the answer as (x+2) (x+3) = x² + 5x + 4

Now, let’s move on to second method

Solving (x+2) (x+3) using FOIL Method

FOIL method is one of the quickest method to simplify the algebraic expressions.

Before that you need to understand the meaning of FOIL. It means

F: Multiple first two terms of each bracket.

O: Multiple outer two terms.

I: Now, multiple inside two terms

L: multiply last two terms.

Then, collect all the like terms and add them.

Let’s solve step by step:

Applying FOIL on (x + 2) (x + 3) means

F: multiplying x from first bracket and x from second bracket. This gives x.x = x².

O: multiplying x from first bracket and 3 from second bracket gives 3x.

I: multiplying 2 from first bracket and x from second bracket gives 2x.

L: on multiplying the last two terms from each bracket gives 2 multiply by 3 = 6

So, we get the four terms as x², 2x, 3x and 6.

Now, It’s time to separate them as per like terms and add them.

x² + (2x + 3x) + 6

Here 2x and 3x are like terms with only one x. There is no more term for   x² and also there is only one constant term i.e. 6.

So, on adding the coefficients of x we get :

= x² + 5x + 4

Whatever method you follow the answer would come out to be the same.

The FOIL method is pretty easy to learn as well as a great way to master the concepts. The more you use this formula, you speed of solving these algebraic terms will amplify.

One another shortcut trick for simplifying such terms is:

Suppose the term is (x +a) (x + b)

You can straightaway use the formula

x² + (a + b)x + ab

In the above equation (x +2) (x +3) we have a =2 and b = 3.

By filling these values in the equation, you get:

x² + (2 + 3)x + 2*3

= x² + 5x + 6

Just memorize this formula and this will not only help you in your GCSE but in future competitive exams as well.

 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

The exact value of sin (75°) is indeed complicated to calculate and searching for the exact answer is troublesome.

But what if you can find it with just a few step procedures? Things get sorted when a complicated equation is divided into two simpler forms and you get the answer easily.

Wondering how? Here is how you can do that. But here’s a pro tip for you. Before moving forward, you must learn the trigno table so that you don’t get stuck just because you don’t know the values.

Book Your 60-minutes Free Trial class NOW!

So, let us start with the equation first of how you can split a value.

Taking the common formula of sin (A+B)

Here this equals to,

sin (A+B) = sin (A) cos (B) + sin (B) cos (A)

Calculating the value of sin (75°)

With the help of this equation, we will find the value of sin (75°)

sin (75°), this can be split as

sin (75°) = sin (30+45) °

sin (75°) = sin (30°) cos (45°) + sin (45°) cos (30°)

So, here we need a few values. Let us first write them to make it easy calculation,

The exact value of sin (30°) = ½

The exact value of cos (45°) = √2/2

The exact value of cos (30°) = √3/2

The exact value of sin (45°) = √2/2

Putting the values in the main equation, and let us find the final value of sin (75°)

sin (75°) = ½ X 1/√2 + (√3)/2 X √2/2

sin (75°) = 1/ (2√2) + (√3)/ (2√2)

sin (75°) = (1 + √3)/ (2√2)

And let us find it in decimal form

After putting the values, the whole equation will proceed like,

sin (75°) = √2/4 + √6/4

sin (75°) = √2 + √6/4

sin (75°) = 0.96592582

Bottom Line!

The outcome of the sin (75°) is easier to calculate when split into two the same way like a complicated equation does help after splitting into the two parts. Therefore, after splitting, as you have seen the value finding was easier. Follow the same steps to find some more values!

Book Your 60-minutes Free Trial class NOW!

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development