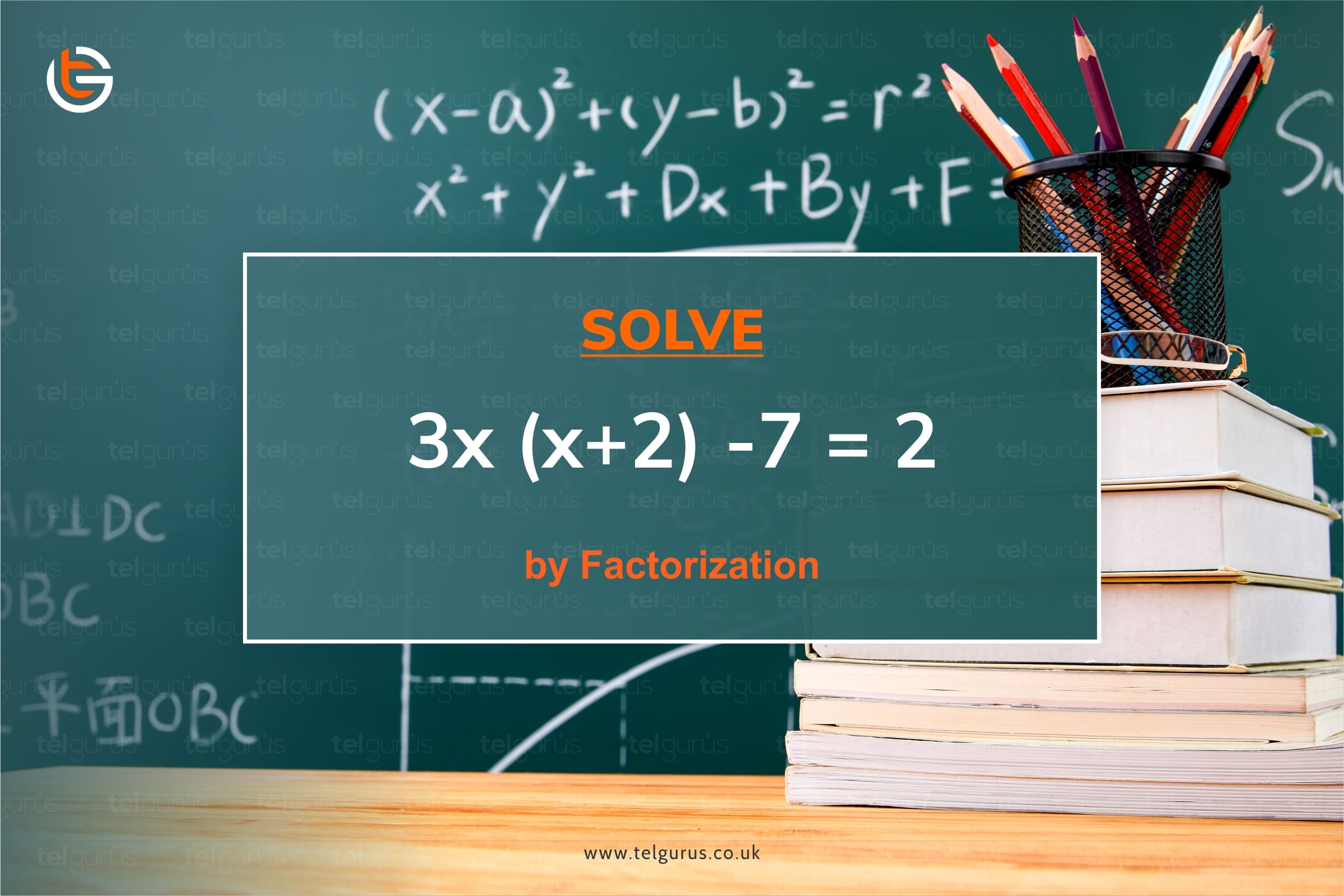Category: Mathematics Questions

Any equation having an algebraic term or variable in the power of two is called quadratic equation.  

Every quadratic equation has one, two or zero roots. These can be real, imaginary or not defined. These roots are also known as zeros of the equation or x-intercepts.  

A quadratic equation is written as    \displaystyle a{{x}^{2}}+bx+c=0

  where a ≠ 0 and is represented by a parabola when the coordinates are plotted on a graph. 

Roots of a quadratic equation   

Solving a quadratic equation means solving the equation for the zeros or roots of the equation.  

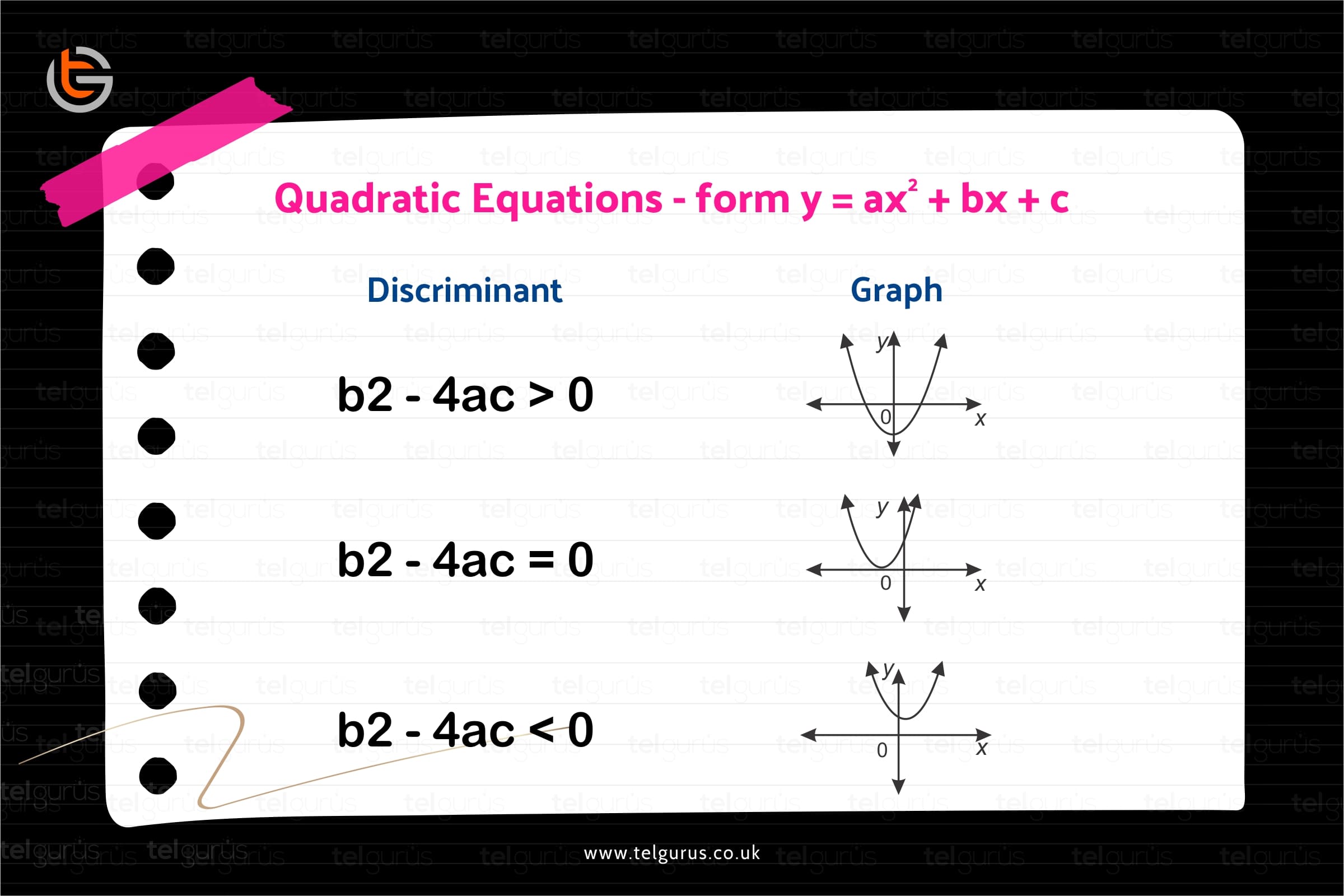
The number and types of roots are determined with the help of Discriminant. 

The roots for any quadratic equation are given by  

x=\frac{{-b~+~\sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}} and x=\frac{{-b-~\sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}}

This formula is also known as quadratic formula. The term \displaystyle {{b}^{2}}-4ac is called the discriminant. 

Discriminant plays an important role in calculating the roots of the equation as it helps in determining how many roots the equation has.    

Three scenarios are there: 

• If b2 – 4ac < 0, then there are no real roots. The roots are undefined.  
• If b2 – 4ac = 0, then there is only one real root.  
• If b2 – 4ac > 0, then the equation has two real roots.  

Lets’ discuss these cases in detail. 

Scenario 1: No Real Roots  

If the discriminate is less than zero which means it is less than zero.  

When plotted on graph, the parabola does not intersect with the x-axis. The negative discriminant means calculating square root of a negative number which is in either case is not defined over the real line.  

For example: let the quadratic equation be \displaystyle {{x}^{2}}-3x+4

D = \displaystyle {{b}^{2}}-4ac = \displaystyle {{(-3)}^{2}}-4*1*4

   = 9 – 16  

   = -7 

The graph of this parabola will never intersect the x-axis and therefore has no real roots.  

Scenario 2: One Real root 

If the D is zero, this means that there is one single point on the graph where the parabola crosses the x-axis.  

To calculate the point, we set \displaystyle {{b}^{2}}-4ac = 0~ in the quadratic formula. The roots become 

x=\frac{{-b\pm \sqrt[{}]{0}}}{{2a}}=\frac{{-b}}{{2a}}

Let’s understand with an example:  

Let, \displaystyle -4{{x}^{2}}+12x-9 = 0~ be the equation 

D =  \displaystyle {{(12)}^{2}}-4*(-4)*(-9)

   = 144 – 144 

  = 0 

As the D is zero, we can determine that this quadratic equation has just one root. 

x = -b/2a = -(12) / 2*4  = -3/2 

Scenario 3: Two real roots 

If D > 0, this means that the equation has two real roots or x-intercepts on the graph.   

The two roots or x-intercepts are defined as  

x1  = \frac{{-b~+~\sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}} and x2 =\frac{{-b-~\sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}}

Let’s take an example and understand the concept: 

Find the roots of \displaystyle 2{{x}^{2}}-11x+5=0

To find the roots, we will first calculate the discriminant i.e. b2 – 4ac 

D = \displaystyle {{(-11)}^{2}}-4*(2)*(5)

   = 121 – 40 

   = 81 

Since D is greater than zero, this means the equation has two real roots which are: 

x1  =\frac{{-\left( {-11} \right)~+~\sqrt[{}]{{81}}}}{{2*2}} = \frac{{11~+~9}}{4} =5

And, x2 = \frac{{-b-~\sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}} = \frac{{11-~~9}}{4} = \frac{1}{2}

So, we can see that the equation has two real and non-zero roots.  

 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

In mathematics, an inverse function is a function that ‘reverses’ another function. 

In other words an inverse function is a function that undoes the action of the function or in simple words we can say it is that function which leaves you to where you started.  

Definition of inverse function 

Inverse of a function agrees with the result, it performs the operation and reaches back to the original function.  

Say, a function ‘f’ maps from x to y, the inverse of ‘f’ will map y to x.  

The function is denoted by ‘f’ or ‘F’, then its inverse is written as \displaystyle {{f}^{{-1}}} or \displaystyle {{F}^{{-1}}}. 

Here -1 does not denotes reciprocal, but is written to show inverse of a function.  

Let f and g be two functions. They are inverse of each other only if f(x) = y and g(y) = 1

Take a real world example to clear the concept.  

Suppose a function is to drive home to the shop then the inverse function will be to drive from shop to back home.  

Basically an inverse function interchanges the first and second elements of each pair of the original function. 

Another example:  

Let a function be f (x) = 3x − 6.   

In this function we have multiplied x by 3 and then subtract 6 from it. 

But, in the reverse function, we will be following the steps backwards. 

So, in the inverse that is now we will first add 6 to undo the subtraction and divide x by 3 to undo the multiplication. 

Symbol to denote inverse of a function f  

 The symbol by which we write inverse of a function is \displaystyle f{{~}^{{-1}}}. 

How to find the inverse of a function? 

Now the question is how to find the inverse of a function.  

Basically there are three methods to find the inverse of a function .  

           1). Simply swapping the ordered pairs 

           2). Solve it algebraically 

           3). Using a graph  

Let’s discuss them in detail. 

1). Finding Inverse  By Swapping  

As the name says, in this method we have to swap the values of x and y. 

Let’s discuss the same with an example. 

Example :  Find the  inverse function  if  f ( x )  =  { ( 4 , 3 )   ( 2 , 1 )  ( 7 , 8 )  ( 6 , 9 ) } 

Since the values of x and y are used only once the function and the inverse function is a one –to– one function. 

A 1-to-1 function means when every second element in the function corresponds to exactly one element. The values of x and y are used only once.   

Therefore the inverse function will be: 

\displaystyle f{{~}^{{-1}}}  =  {  (3 , 4 )  ( 1 , 2 )  ( 8 , 7 )  (9 , 6 )  } 

So we can see that we have just interchanged the elements to find the inverse of a function. This is known as swapping of elements.  

2).  Finding Inverse Algebraically  

To find inverse algebraically we have to follow three steps.  

Step 1: Set the function as y 

Step 2: Swap the variables x and y  

Step 3: Solve y  

Example :  

f ( x )  = x –  4

Sol:    Let  y = x – 4          (step 1) 

                    x = y – 4          (Step 2) 

                    x + 4 = y           (Step 3) 

Therefore   

\displaystyle f{{~}^{{-1}}} =  x + 4

3). Using a graph 

The general method of finding the inverse of a function using a graph is to swap the coordinates of x and y. 

But this is merely a relation and is not necessarily needs to be a function. 

To apply graphs, you need to ensure that the original function needs to be a one-to-one function so that its inverse is also a function.  

For a one-to-one function F, graphs of F and \displaystyle F{{~}^{{-1}}} are reflection of each other on the line  y=x.  

Also, the range of \displaystyle F{{~}^{{-1}}}  is the domain of F and vice versa.

 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

We know that an equation consists of an algebraic expression which is equated to zero.  

The algebra formulas are just not used to solve these equations but form the foundation of various mathematical concepts.  

Many topics such as quadratic equations, coordinate geometry, polynomials etc use these formulas for solution.  These formulas help in simplifying the expressions and deducing the answers.  

Before moving on to formulas, first understand what is Algebra and what are algebraic expressions.  

What is Algebra?

Algebra is a more like a generalized arithmetic where letters are substituted for numbers.  

The letters are known as variables and they don’t have a fixed value. The numbers are called constants as they have a fixed value.    

Algebra is a wide branch of mathematics that covers vectors, matrices, complex numbers etc. 

x, y, a and b are common letters that are used to represent the variables. 

What is algebraic expression?

A combination of variables and constants which is connected with each other through various arithmetic operators such as +, -, x or ÷ is known as algebraic expression.  

Basic Algebraic formulas to solve equations

• • \displaystyle {{\left( {a+b} \right)}^{2}}~=~{{a}^{2}}~+~2ab~+~{{b}^{2}}
  • \displaystyle {{\left( {a-b} \right)}^{2}}~=~{{a}^{2}}~-~2ab~+~{{b}^{2}}
  • \displaystyle {{a}^{2}}-{{b}^{2}}=(a+b)(a-b)
  • \displaystyle {{a}^{2}}~+~{{b}^{2}}~=~{{\left( {a~+~b} \right)}^{2}}-2ab
  • \displaystyle {{\left( {a~+~b~+~c} \right)}^{2}}~=~{{a}^{2}}~+~{{b}^{2}}~+~{{c}^{2}}~+~2ab~+~2bc+2ca
  • \displaystyle {{\left( {a~-~b~-~c} \right)}^{2}}~=~{{a}^{2}}~+~{{b}^{2}}~+~{{c}^{2}}~-2ab~+~2bc-2ca
  • \displaystyle {{(a+b)}^{3}}={{a}^{3}}+3{{a}^{2}}b+3a{{b}^{2}}+{{b}^{3}}={{a}^{3}}+{{b}^{3}}+3ab(a-b)
  • \displaystyle {{(a-b)}^{3}}={{a}^{3}}-3{{a}^{2}}b+3a{{b}^{2}}-{{b}^{3}}={{a}^{3}}-{{b}^{3}}-3ab(a-b)
  • \displaystyle {{a}^{3}}-{{b}^{3}}=(a-b)({{a}^{2}}+ab+{{b}^{2}})
  • \displaystyle {{a}^{3}}+{{b}^{3}}=(a+b)({{a}^{2}}-ab+{{b}^{2}})
  • \displaystyle {{(a+b)}^{4}}={{a}^{4}}+4{{a}^{3}}b+6{{a}^{2}}{{b}^{2}}+4a{{b}^{3}}+{{b}^{4}}
  • \displaystyle {{(a-b)}^{4}}={{a}^{4}}-4{{a}^{3}}b+6{{a}^{2}}{{b}^{2}}-4a{{b}^{3}}+{{b}^{4}}
  • \displaystyle {{a}^{4}}-{{b}^{4}}=(a-b)(a+b)({{a}^{2}}+{{b}^{2}})
  • \displaystyle {{a}^{{-m}}}=\frac{1}{{{{a}^{m}}}}

Formulas involving exponents

Let’s have a look at some very commonly used formulas for expressions having same base but different powers. 

• • \displaystyle {{a}^{m}}.{{a}^{n}}={{a}^{{m+n}}}
  • \displaystyle \frac{{{{a}^{m}}}}{{{{a}^{n}}}}={{a}^{{m-n}}}
  • \displaystyle {{({{a}^{m}})}^{n}}={{a}^{{mn}}}
  • \displaystyle {{\left( {ab} \right)}^{n}}={{a}^{n}}.{{b}^{n}}
  • \displaystyle {{a}^{0}}=1
  • \displaystyle {{a}^{{-m}}}=\frac{1}{{{{a}^{m}}}}

Roots of quadratic equation

To find the roots of a quadratic equation of the form \displaystyle a{{x}^{2}}+~bx+c=0, you can use the following formula: 

\displaystyle x=~\frac{{-b\pm \sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}}

Here,  \displaystyle {{b}^{2}}-~4ac is known as discriminant or D. 

D is helpful in finding the nature of roots of the equation.  

• If D > 0, then the roots of the quadratic equation will have two real and distinct roots.   
• If D = 0, then the two roots of the quadratic equation will be equal and real. 
• If D < 0, then the quadratic equation will have two imaginary roots.  

Let’s apply these using some examples: 

Q1. Solve the expression \displaystyle {{25}^{2}}-~{{14}^{2}}

Solution:

For this question, no need to calculate the squares of the number. Just you need to apply the formula listed above. 

Using \displaystyle {{a}^{2}}-~{{b}^{2}}=\left( {a-b} \right)\left( {a+b} \right)

We can write as: 

\displaystyle {{25}^{2}}-~{{14}^{2}}=\left( {25-14} \right)\left( {25+14} \right)

=11*39

=329

Q2. Solve the expression:   \displaystyle {{5}^{2}}{{.5}^{3}}

Solution: By using the formula      \displaystyle {{a}^{m}}.{{a}^{n}}={{a}^{{m+n}}}

\displaystyle {{5}^{2}}{{.5}^{3}}={{5}^{{2+3}}}={{5}^{5}}=3125

 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

Trigonometry has three most important functions which are primarily used by the students to find out the unknown value of an angle and the length of sides of a right angled triangle.  The names and abbreviations of these three functions are sine (sin), cosine (cos) and Tangent (tan). 

These three are the primary functions and there are other three trigonometric functions which are the reciprocals of these functions.  

Cosecant (cosec) is the reciprocal of sine, Secant (sec) is the reciprocal of cosine and Cotangent (cot) is the reciprocal of tangent.   

The values of these functions are used in trigonometric equations. 

But the question is how to remember the values of these functions. 

There is a table of the values of the trigonometric functions but remembering all the values from the table is a daunting task. 

 First we will discuss the simple method by means of which we can calculate the value of sine ratios for all the degrees. 

With this method, you can easily calculate the values for all other trigonometry ratios. 

Read More – Mathematics Questions

There are mainly five angles that you need to focus, these are 0°, 30°, 45°, 60° and 90°. 

sin 0° = √ (0 / 4) = 0 

sin 30° = √ (1 / 4) = 1 / 2  

sin 45° = √ (2 / 4) = 1 / √ 2  

sin 60° = √ (3 / 4) = √ 3 / 2  

sin 90° = √ (4 / 4) = 1  

So, in this way we can find the values of sine function. 

 The values of cosine or cos functions can be obtained by writing the values in the opposite direction.  Let’s see how to learn them: 

cos 0° = √ (4 / 4) = 1 

cos 30° = √ (3 / 4) = √ 3 / 2  

cos 45° =√ (2 / 4) =  1 / √ 2  

cos 60° = √ (1 / 4) = 1 / 2  

cos 90° = √ (0 / 4) = 0  

This concludes that, the values of cos function are in the opposite direction of sine functions 

sin 0° = cos 90° 

sin 30° = cos 60°  

sin 45° = cos 45°  

sin 60° = cos 30°  

sin 90° = cos 0°  

Now, Let’s move to tangent functions  

Tangent of an angle theta (θ) is obtained by dividing sin of that angle by cos of the same angle.  

This means, tan θ = sinθ / cosθ 

Therefore we can find the values of tan functions with the help of values of sin and cos functions.  

This comes out to be:  

 Tan 0° = sin 0° / cos 0° = 0 / 1 = 0  

Tan 30° = sin 30° / cos 30°   = ( 1 / 2 )   /  ( √ 3 / 2 )  =  1 / √ 3  

Tan 45° = sin 45° / cos 45° = ( 1 / √ 2 )   /  ( 1 / √ 2 ) = 1 

Tan 60° =  sin 60°  / cos 60°  =  ( √ 3 / 2 )  /  ( 1 / 2 ) =  √ 3 

Tan 90° = sin 90° / cos 90° = 1 / 0   = ∞   that means not defined or infinity. 

Similarly, we can find the values of cosec functions by taking the reciprocals of respective sine function, values of secant functions by taking the reciprocals of cosine functions and that of cotangent by the reciprocals of tangent function. 

 So, we can see that just by finding the values of sine function we can easily find the values of other trigonometric functions. 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

Just like other concepts, Pythagoras theorem has its own relevance in mathematics. It explains the relation between the sides of the right-angled triangle.  This theorem was invented by famous Greek mathematician named “Pythagoras”.  

This formula states the relationship between base, perpendicular and hypotenuse of a right angled triangle. Let’s discuss the same in detail.  

Pythagoras Theorem  

According to Pythagoras Theorem, “In a right angled triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides which are knows as base and perpendicular”. 

Let’s us understand the concept. 

 As we know that in a right triangle we have three sides Hypotenuse, perpendicular and base. The side that is opposite to the 90 degree angle is called the hypotenuse which is also the longest side.   

So, according to Pythagoras theorem: 

\displaystyle (\text{ }Hypotenuse~){{~}^{2}}~~~=~~\text{ }{{\left( {\text{ }Base\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }Perpendicular\text{ }} \right){{~}^{2}}

In the above right triangle ABC  right angled at B  ,  side AC is the hypotenuse (the longest side),  side BC serves as the base and side AB  is  the perpendicular.  

The base and perpendicular can be used interchangeably.  

So, according to Pythagoras Theorem  

\displaystyle (\text{ }AC~){{~}^{2}}~~~=~~\text{ }{{\left( {\text{ }BC\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }AB\text{ }} \right){{~}^{2}}

So, this Pythagorean Theorem builds a relationship between all the three sides of a right angled triangle. 

We can use this theorem to find the unknown side of a triangle when two of its sides are given.   

Let us take some examples: 

Example 1

Question. Find the hypotenuse of a right triangle if the base is  3 cm  and  perpendicular is  4 cm ? 

Solution:  According to Pythagoras theorem 

(\text{ }Hypotenuse~){{~}^{2}}~~~=~~\text{ }{{\left( {\text{ }Base\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }Perpendicular\text{ }} \right){{~}^{2}}

                                          =~~\text{ }{{\left( {\text{ }3\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }4\text{ }} \right){{~}^{2}}

                                          = 9  +   16  

                                          = 25 

Hypotenuse  =  √ 25 

 Hypotenuse   =  5  

Therefore , Hypotenuse of the triangle is  5 cm . 

Example 2

Question: Find the base of a right triangle if the hypotenuse of the triangle is 17 cm and the perpendicular is 15 cm? 

Solution:  As it’s a right angled triangle, we can Apply Pythagoras theorem  

\displaystyle (\text{ }Hypotenuse~){{~}^{2}}~~~=~~\text{ }{{\left( {\text{ }Base\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }Perpendicular\text{ }} \right){{~}^{2}} 

By  putting the  given values   in the above relation we get , 

\displaystyle (\text{ }17~){{~}^{2}}~~~=~~\text{ }{{\left( {\text{ }Base\text{ }} \right)}^{2}}~~\text{ }+\text{ }\left( {\text{ }15\text{ }} \right){{~}^{2}} 

 

\displaystyle ~~~~~\text{ }289~~~~~~~~=~\text{ }(~Base\text{ })~2~\text{ }+~\text{ }225~

 

\displaystyle ~289~\text{ }-~\text{ }225~\text{ }=~\text{ }\left( {\text{ }Base\text{ }} \right){{~}^{2}}

 

\displaystyle 64~~~~~~=~\text{ }(~Base\text{ }){{~}^{2}}

  Base  =  √ 64

 Base  =  8  

Therefore  , the base of the triangle is 8 cm . 

So, this is how we can use the Pythagoras theorem to find out the unknown side of the right triangle. This theorem is not only used in geometry, this is also used in real–life scenarios as well.  

Real Life Applications of Pythagoras Theorem  

•  We can use Pythagoras theorem to check whether a triangle is a right triangle or not.   

• In ocean studies, Pythagorean formula is used to calculate the speed of the sound waves in water/sea.  
• Metrological department and aerospace industry uses this theorem to determine the sound source and its range.  
• In navigation this theorem is used to find the shortest distance between given points.  
• In construction, architecture and planning, this theorem is used to calculate the slope of roof, dam work, drainage system, etc .  

 

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

In the question what we have to solve is the equation. In algebra, an equation can be defined as a mathematical statement which contains an equal symbol between two algebraic expressions that have same value.   

In mathematics, the most basic and common algebraic equations consist of one or more variables.  

For Example: