Enrich your knowledge with our informative blogs
What is an Inverse function?
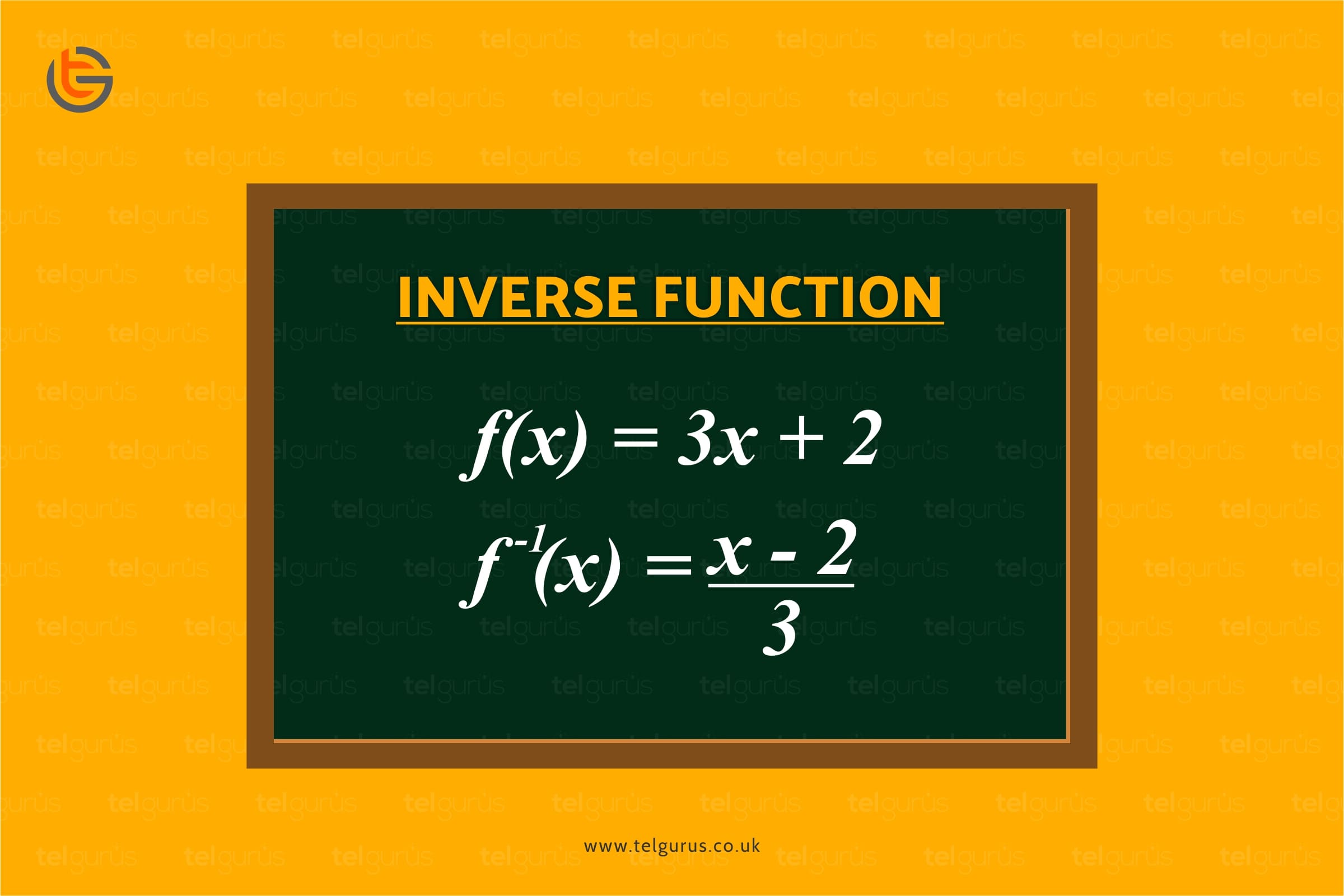
In mathematics, an inverse function is a function that ‘reverses’ another function.
In other words an inverse function is a function that undoes the action of the function or in simple words we can say it is that function which leaves you to where you started.
Definition of inverse function
Inverse of a function agrees with the result, it performs the operation and reaches back to the original function.
Say, a function ‘f’ maps from x to y, the inverse of ‘f’ will map y to x.
The function is denoted by ‘f’ or ‘F’, then its inverse is written as \displaystyle {{f}^{{-1}}} or \displaystyle {{F}^{{-1}}}.
Here -1 does not denotes reciprocal, but is written to show inverse of a function.
Let f and g be two functions. They are inverse of each other only if f(x) = y and g(y) = 1
Take a real world example to clear the concept.
Suppose a function is to drive home to the shop then the inverse function will be to drive from shop to back home.
Basically an inverse function interchanges the first and second elements of each pair of the original function.
Another example:
Let a function be f (x) = 3x − 6.
In this function we have multiplied x by 3 and then subtract 6 from it.
But, in the reverse function, we will be following the steps backwards.
So, in the inverse that is now we will first add 6 to undo the subtraction and divide x by 3 to undo the multiplication.
Symbol to denote inverse of a function f
The symbol by which we write inverse of a function is \displaystyle f{{~}^{{-1}}}.
How to find the inverse of a function?
Now the question is how to find the inverse of a function.
Basically there are three methods to find the inverse of a function .
1). Simply swapping the ordered pairs
2). Solve it algebraically
3). Using a graph
Let’s discuss them in detail.
1). Finding Inverse By Swapping
As the name says, in this method we have to swap the values of x and y.
Let’s discuss the same with an example.
Example : Find the inverse function if f ( x ) = { ( 4 , 3 ) ( 2 , 1 ) ( 7 , 8 ) ( 6 , 9 ) }
Since the values of x and y are used only once the function and the inverse function is a one –to– one function.
A 1-to-1 function means when every second element in the function corresponds to exactly one element. The values of x and y are used only once.
Therefore the inverse function will be:
\displaystyle f{{~}^{{-1}}} = { (3 , 4 ) ( 1 , 2 ) ( 8 , 7 ) (9 , 6 ) }
So we can see that we have just interchanged the elements to find the inverse of a function. This is known as swapping of elements.
2). Finding Inverse Algebraically
To find inverse algebraically we have to follow three steps.
Step 1: Set the function as y
Step 2: Swap the variables x and y
Step 3: Solve y
Example :
f ( x ) = x – 4
Sol: Let y = x – 4 (step 1)
x = y – 4 (Step 2)
x + 4 = y (Step 3)
Therefore
\displaystyle f{{~}^{{-1}}} = x + 4
3). Using a graph
The general method of finding the inverse of a function using a graph is to swap the coordinates of x and y.
But this is merely a relation and is not necessarily needs to be a function.
To apply graphs, you need to ensure that the original function needs to be a one-to-one function so that its inverse is also a function.
For a one-to-one function F, graphs of F and \displaystyle F{{~}^{{-1}}} are reflection of each other on the line y=x.
Also, the range of \displaystyle F{{~}^{{-1}}} is the domain of F and vice versa.
Read More – Mathematics Questions
View More – Useful links for Your Child’s Development

Unleash the Power of visualization to break tough concepts
Wanna be the next Maths wizard? Discover the new way of learning concepts with real-life Visualization techniques and instant doubt resolutions.
Categories
Recent Posts
- List of the qualities you should look for in your tutors?
- What is the most useful formulas in math?
- Describe the process of eating to defecation of food?
- Difference between the natural and artificial active response by the immunology system.
- Explain the different circle theorems
- How are nerve cells adapted to their function?