Explain the different circle theorems

• Book A Demo Class

A circle is the locus of all the equidistant points from a particular fixed point.

This fixed point is referred to as the centre of a circle.

The circle theorem includes the concepts of angles, sectors, tangents, circle chords, and proofs.

Let us learn about the circle theorems here only. 

Circle Theorems 

The different circle theorems include: 

__________________________________________________________________

Theorem 1

“Two equal chords of the circle subtend equal angles at the circle’s centre.”

__________________________________________________________________

Proof of the theorem: 

In ∆AOB and ∆COD,

AB=CD {because both have equal chords} ————- (1)

OA= OB= OC= OD {Circle Radii} —————- (2)

From the equation (1) and (2),

∆AOB ≅ ∆COD

Therefore by CPCT, we get

∠AOB = ∠COD

Hence Proved. 

__________________________________________________________________

The Converse of Theorem 1

“If two angles subtended at the centre by two chords are equal, the chords are also of equal lengths.”

__________________________________________________________________

[REFERRING TO THE SAME IMAGE USED IN THE FIRST THEOREM] 

In ∆AOB and ∆COD,

∠AOB = ∠COD (Equal angles subtended at the circle’s centre “O”) ———– (1)

OA= OB= OC= OD ———— (2)

From equations 1 and 2,

∆AOB ≅ ∆COD

Therefore, By CPCT, we get AB = PQ. 

__________________________________________________________________

THEOREM 2

“The Perpendicular to a chord bisects the chord if drawn from the centre of a circle.”

__________________________________________________________________

According to the theorem, in the figure OP ⊥ AB.

Therefore, AP = PB 

Proof of the theorem 

In ∆AOP and ∆BOP,

∠APO = ∠BPO = 90° (OP ⊥ AB) ———— (1)

OA = OB —— (2)

OP = OP (COMMON SIDES) ——— (3)

From the equations 1, 2, and 3,

AP = PB By CPCT

__________________________________________________________________

The converse of the Theorem 2

“A straight line passing through a circle’s centre to bisect the chord is perpendicular to the chord.”

__________________________________________________________________

REFERRING THE SAME IMAGE USED IN THEOREM 2 

In ∆AOP and ∆ BOP,

AP = PB (As OP bisects AB) ———- (1)

OA = OB (Radii of circle) ————- (2)

OP = OP (Common side) ————— (3)

From equations 1, 2, and 3,

∠APO = ∠BPO = 90° (By CPCT) 

__________________________________________________________________

Theorem 3

“Equal chords of a circle are equidistant from the circle’s center.”

__________________________________________________________________

Construction: Join OB and OD 

[REFERENCE IMAGE ONLY: Using EF instead of PQ] 

Proof of Theorem 

In ∆OEB and ∆OFD,

BE = ½ AB (Perpendicular to a chord bisects it) ——— (1)

DF = ½ CD ——————— (2)

Given, AB = CD

BE = DF (from equations 1 and 2)

OB = OD (Radii of the same circle)

∠OEB = ∠OFD = 90°

∆OEB ≅ ∆OFD

Hence, OE = OF (By CPCT) 

__________________________________________________________________

The converse of the 3rd Theorem

“Chords of a circle that are equidistant from the centre are equal in length.”

__________________________________________________________________

REFERRING THE SAME IMAGE USED IN THIRD THEOREM 

In ∆OEB and ∆OFD,

OE = OF —————– (1)

∠OEB = ∠OFD = 90° ———– (2)

OB = OD ———– (3)

From equations 1, 2 and 3

∆OEB ≅ ∆OFD

BE = FD (By CPCT)

½ AB = ½ CD

Hence, AB = CD 

__________________________________________________________________

Theorem 4

“Measures of angles subtended to any point on the circle’s circumference from the same arc are equal to half of the angle subtended at the centre by the same arc.” __________________________________________________________________

[REFERENCE IMAGE ONLY: Using Q instead of D] 

In ∆AOP,

∠AOB = 2∠APB

Construction required: Joining PQ passing through the “O” 

Poof of the theorem 

OA = OP ————— (1)

∠OAP = ∠OPA (Angles opposite to equal sides of triangle) ——————— (2)

∠AOQ = ∠OAP + ∠OPA ——————- (3)

Therefore, from equations 2 and 3

∠AOQ = 2∠OPA ———- (4)

In ∆BOP,

∠BOQ = 2∠OPB ——— (5)

∠AOB = ∠AOQ + ∠BOQ

From equations 4 and 5,

∠AOB = 2∠OPA + 2∠OPB

∠AOP = 2 (∠OPA + ∠OPB)

∠AOB = 2∠APB

Hence proved

__________________________________________________________________

Theorem 5

“The opposite angles in a cyclic quadrilateral are supplementary.”

__________________________________________________________________ 

[REFERENCE IMAGE ONLY: Using PQRS instead of ABCD] 

Proof of the theorem 

For arc PQR,

∠POR = 2 ∠PQR = 2α (THEOREM 4) ————— (1)

Considering the arc PSR,

Reflex ∠POR = 2 ∠PSR = 2β (THEOREM 4) —————- (2)

∠POR + Reflex ∠POR = 360°

From equations 1 and 2

2 ∠PQR + 2 ∠PSR = 360°

2α + 2β = 360°

α + β = 180° 

__________________________________________________________________

Theorem 6

“Tangent to a circle perpendicular to the radius of the circle at the point of contact”

__________________________________________________________________

 [REFERENCE IMAGE ONLY: Using AB instead of PQ]

Here in this diagram,

O is the centre of the circle.

OA ⊥ XY. 

__________________________________________________________________

Theorem 7

“Number of tangents can be drawn from a given point.”

__________________________________________________________________

(1) If the point is the circle’s interior region, any line through that particular point will be a secant. Therefore, no tangent can be drawn to a circle that passes through the point that lies inside it.

[REFERENCE IMAGE ONLY]

(2) When the tangency point lies on a circle, there is precisely one tangent to a circle, which passes through it.

[REFERENCE IMAGE ONLY]

(3) When the point is at the circle’s outside, there are precisely two tangents to the circle.

[REFERENCE IMAGE ONLY] 

__________________________________________________________________

Theorem 8

“The length of the tangents drawn from any external point is equal.”

__________________________________________________________________

[REFERENCE IMAGE ONLY]

Discover better learning pace and Flexibility

Online tuition by TEL Gurus helps you learn at your own pace and garner the flexibility to choose your preferred timeslots.

Book A Demo Class

• 

  How are nerve cells adapted to their function?

• 

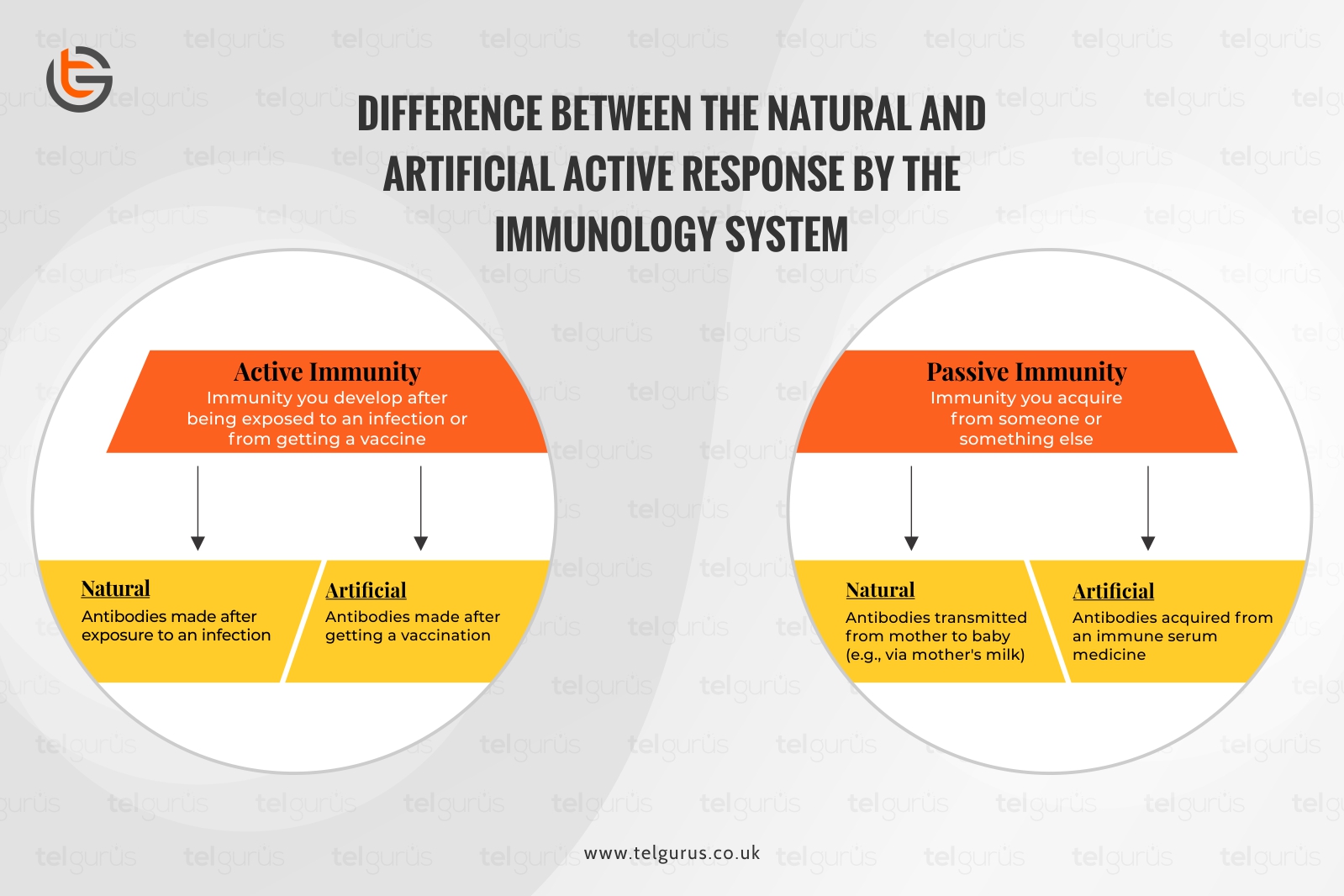
  Difference between the natural and artificial active response by the immunology system.