Integrals in mathematics play an important role in calculating many useful quantities such as areas, volumes, displacement etc. When we talk about integrals we usually talk about definite integrals.
When the upper and lower limits of integration are given as constants, it is termed as definite integral.
On the other hand, in indefinite integral no limits of integration are there.
The indefinite integrals are used for the calculations of anti-derivatives.
Calculation of integrals is known as integration. Integration is one of the two important calculus topics in mathematics apart from differentiation.
Integration is a very vast topic. Integration is a way of adding small parts to find the whole.
It’s is like a whole pizza and the pieces of it are the differentiable functions which can be integrated. The concept of integration has been developed to solve the following types of problems:
- To solve the problem function, when its derivatives are given.
- To calculate the area bounded by the graph of a function under certain constraints or limits.
In maths, integration is a method of adding or summing up the parts to find the whole. So, integration and differentiation are the inverse of each other.
The symbol used for integration is ∫.
For Example:
We know that the
cosx is the derivative of x.
So, sin x is the antiderivative or integral of x.
So, integration is a reverse process of differentiation. Integration of a constant function is x dx.
Calculation of small addition problems is an easy task which we can do manually or by using calculators as well. But for big addition problems where the limits could reach to infinity, integration methods are used .
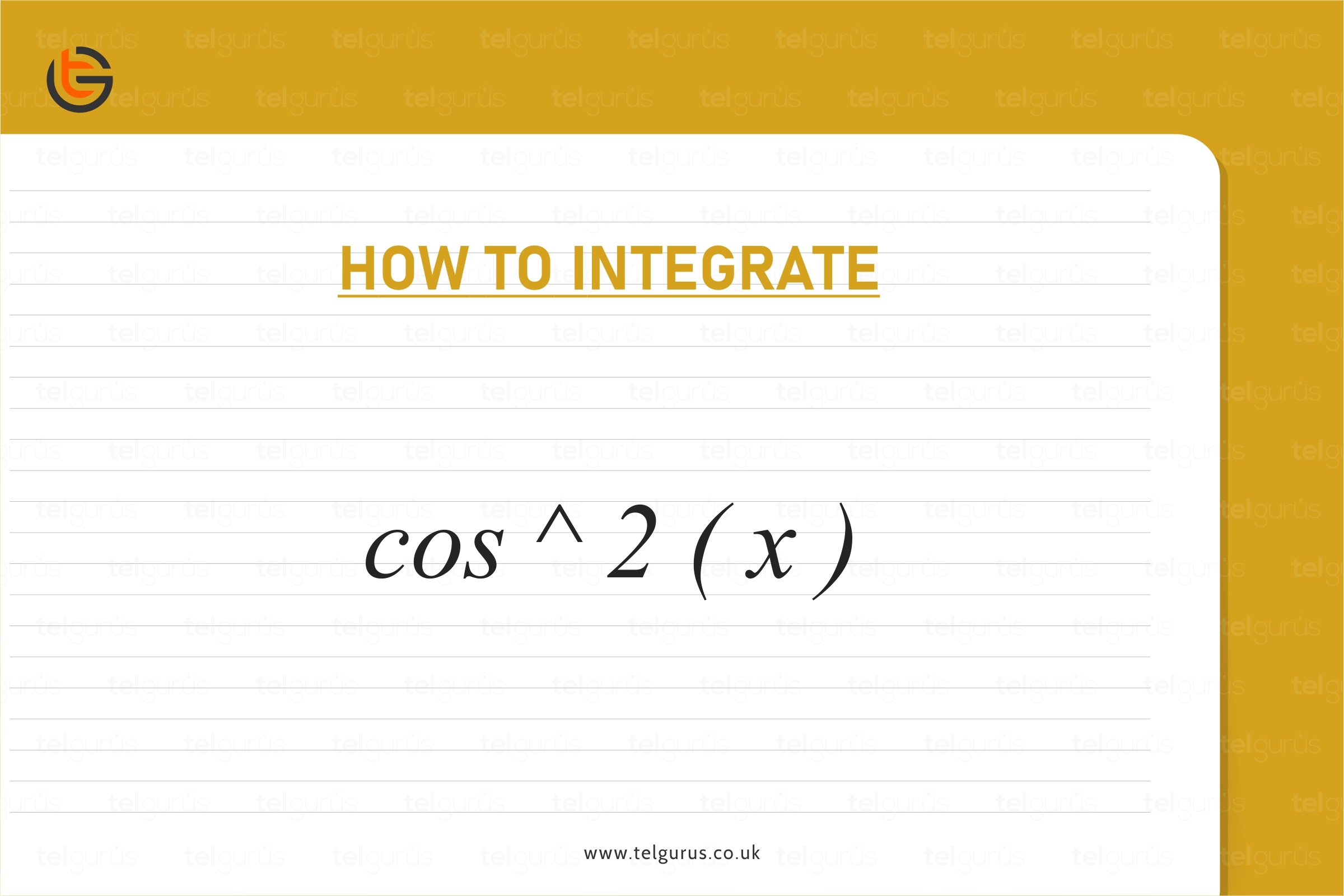
Now we need to find the integral of \displaystyle {{\cos }^{2}}x and \displaystyle cos~\hat{\ }2\left( x \right)?
Let the integration is of the form
\displaystyle I~=~~\int ~~cos~2~\left( {~x~} \right)~~dx
The integral cannot be evaluated by the direct formula of integration.
So, using the trigonometric formulas and identity functions:
We know that, \displaystyle \cos 2\text{x}={{\cos }^{2}}x-{{\sin }^{2}}x …………………………………….(i)
\displaystyle {{\cos }^{2}}x+{{\sin }^{2}}x=1 …………………………………….. (ii)
Taking the value of \displaystyle {{\sin }^{2}}x~ from (ii) and replacing in (i), we get,
\displaystyle \cos 2\text{x}={{\cos }^{2}}x-(1-co{{s}^{2}}x)\displaystyle \cos 2\text{x}={{\cos }^{2}}x-1+{{\cos }^{2}}x
\displaystyle \cos 2\text{x}=2{{\cos }^{2}}x-1
so, \displaystyle {{\cos }^{2}}x=\frac{{\left( {\cos 2x~+1} \right)}}{2} …………………(iii)
We will use this value of \displaystyle {{\cos }^{2}}x and put in our original integration equation.
\displaystyle \int{{{{{\cos }}^{2}}xdx}} = \displaystyle \int{{\frac{{\cos 2x+1}}{2}dx}} = \displaystyle \int{{(\frac{{\cos 2x}}{2}+\frac{1}{2})dx}}
= \displaystyle \int{{\frac{{\cos 2x}}{2}dx+\int{{}}\frac{1}{2}dx}}
= \displaystyle \frac{{sin2x}}{2}*\frac{1}{2}~+\frac{x}{2}+~c [We know that integral of \displaystyle \int{{\sin xdx=\cos x}} ]
= \displaystyle \frac{{sin2x}}{4}+\frac{x}{2}+~c
So, the integral of \displaystyle \int{{{{{\cos }}^{2}}xdx}} becomes \displaystyle ~\frac{{sin2x}}{4}+\frac{x}{2}+~c. c is the constant here.
For all indefinite integrals we have to add an arbitrary constant (c). This constant can take the value of any real number.
Read More – Mathematics Questions
View More – Useful links for Your Child’s Development