Enrich your knowledge with our informative blogs
Discriminants and determining the no. of real roots of a quadratic equation.
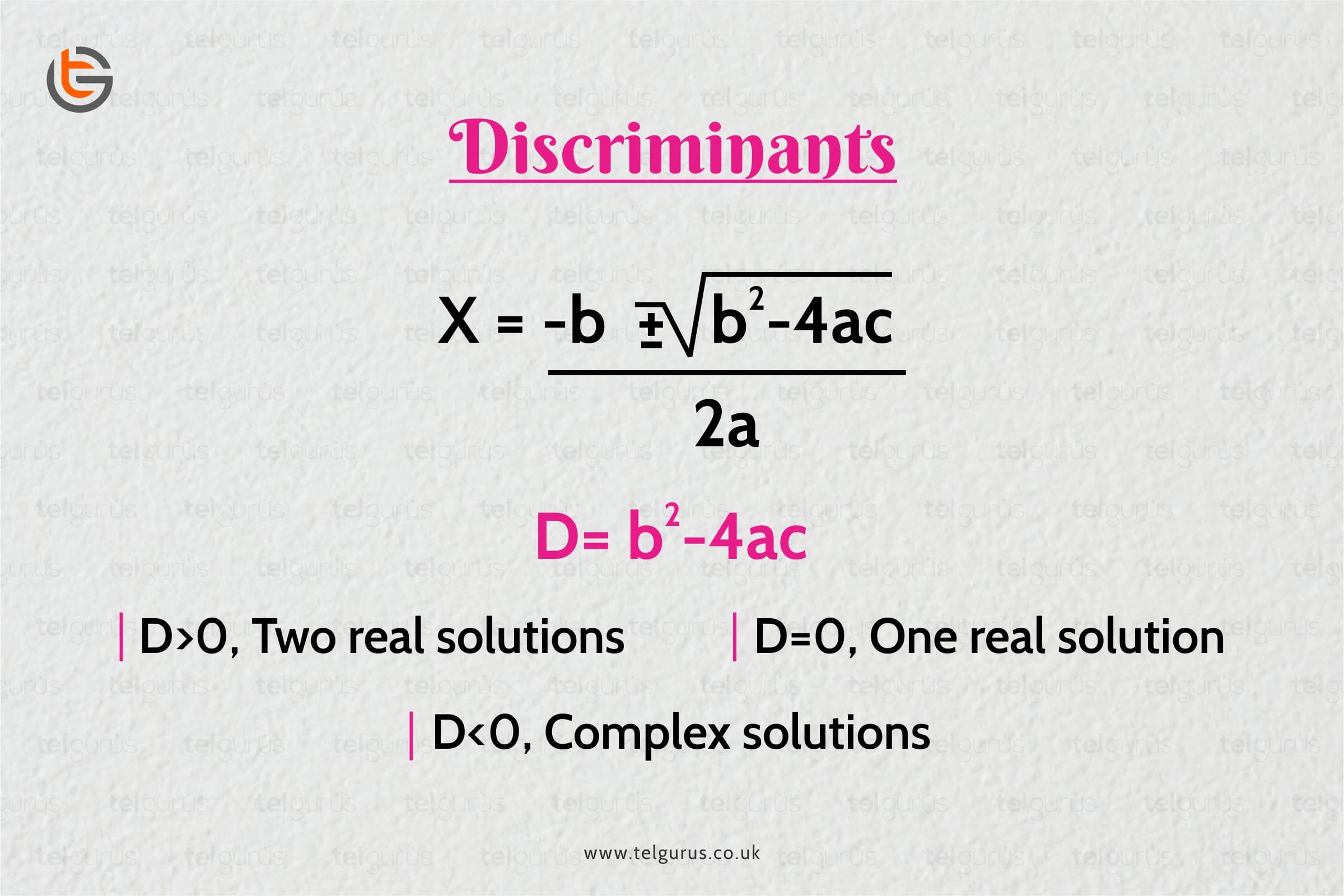
What is discriminant in math?
Discriminant in math is a function of the coefficients of the polynomial. Discriminant Symbol
The symbol of discriminant is “D“.
To find the number of real roots of quadratic equation first we should know what is the quadratic equation?
A quadratic equation is that in which the maximum power of variable is 2
General Form of Quadratic Equation
A quadratic equation is in the form: \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~
The discriminant of a quadratic equation \displaystyle ~a{{x}^{2}}~+~bx~+~c~ is in terms of a , b , and c is
\displaystyle D~=~{{b}^{2}}-~~4acLet us clear the concept of discriminant with help of some examples:
Example1
Find the discriminant of \displaystyle ~2{{x}^{2}}~+~3x~+~3~=~0~
Sol: Compare the given expression with \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~
a=2
b=3
c=3
The discriminant of the given equation is
By applying the formula \displaystyle D~=~{{b}^{2}}-~~4ac
= \displaystyle ~{{3}^{2}}~-~4(2)(3)~
= 9 – 24
= − 15
Thus, the discriminant of the given equation is − 15
There are three cases for the discriminant:
Case 1:
\displaystyle ~{{b}^{2}}~-~4ac~>~0~
If the discriminant is greater than zero, this means that the quadratic equation has two real, distinct roots.
Let’s understand that with an example:
\displaystyle ~{{x}^{2}}~-~5x~+~2~=~0~
Here, a = 1 , b= – 5 , c= 2
Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac
= \displaystyle ~{{(-5)}^{2}}~-~4(1)(2)~
= 17
Discriminant is greater than zero i.e. 17 > 0.
Therefore, the roots of the above equation will be real and distinct.
Case 2:
When, \displaystyle ~{{b}^{2}}~-~4ac~<~0~
If the discriminant is less than zero then the quadratic equation has no real roots.
Example:
\displaystyle ~3{{x}^{2}}~-~2x~+~1~=~0~
Here, a= 3 , b = 2 , c= 1
Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac
= \displaystyle ~{{(2)}^{2}}~-~4(3)(1)~
= 4 − 12
= − 8 which is < 0
In above equation value of the discriminant is less than zero. Therefore, the roots of this quadratic equation are not real.
Case 3:
When, D or \displaystyle ~{{b}^{2}}~-~4ac~=~0~
If the discriminant is equal to zero, this means that the quadratic equation has two real, identical roots .
Example :
\displaystyle ~{{x}^{2}}~+~2x~+~1~=~0~
Comparing the above equation with general quadratic equation \displaystyle ~a{{x}^{2}}~+~bx~+~c~=~0~
We get a = 1, b = 2, c = 1
Discriminant , \displaystyle D~=~{{b}^{2}}-~~4ac
= \displaystyle ~{{(2)}^{2}}~-~4(1)(1)~
= 4 − 4
= 0
In the above equation discriminant is zero therefore the above equation has two real, identical roots.
So, from above discussion we can conclude that
-
- If discriminant < 0, there are 2 imaginary roots . Imaginary roots are those which are in the form of i which is known as ita in mathematics as discriminant is negative.
-
- If discriminant > 0, there are 2 real, distinct roots.
- If discriminant = 0, there is one real root and the value of that root is \frac{{-b}}{{2a}}
So, the discriminant of a quadratic polynomial provides information about the properties of the roots of the polynomial.
Read More – Mathematics Questions
View More – Useful links for Your Child’s Development

Unleash the Power of visualization to break tough concepts
Wanna be the next Maths wizard? Discover the new way of learning concepts with real-life Visualization techniques and instant doubt resolutions.
Categories
Recent Posts
- List of the qualities you should look for in your tutors?
- What is the most useful formulas in math?
- Describe the process of eating to defecation of food?
- Difference between the natural and artificial active response by the immunology system.
- Explain the different circle theorems
- How are nerve cells adapted to their function?