How do you change the subject of the formula?

• Book A Demo Class

Every expression in algebra or a formula comprises of more terms, variables and constants.  

The main subject is always kept on the right hand side and all the dependent variables are kept on left hand side.  

The values for the subject are calculated by substituting the values in right hand side of the expression. 

 For example:  

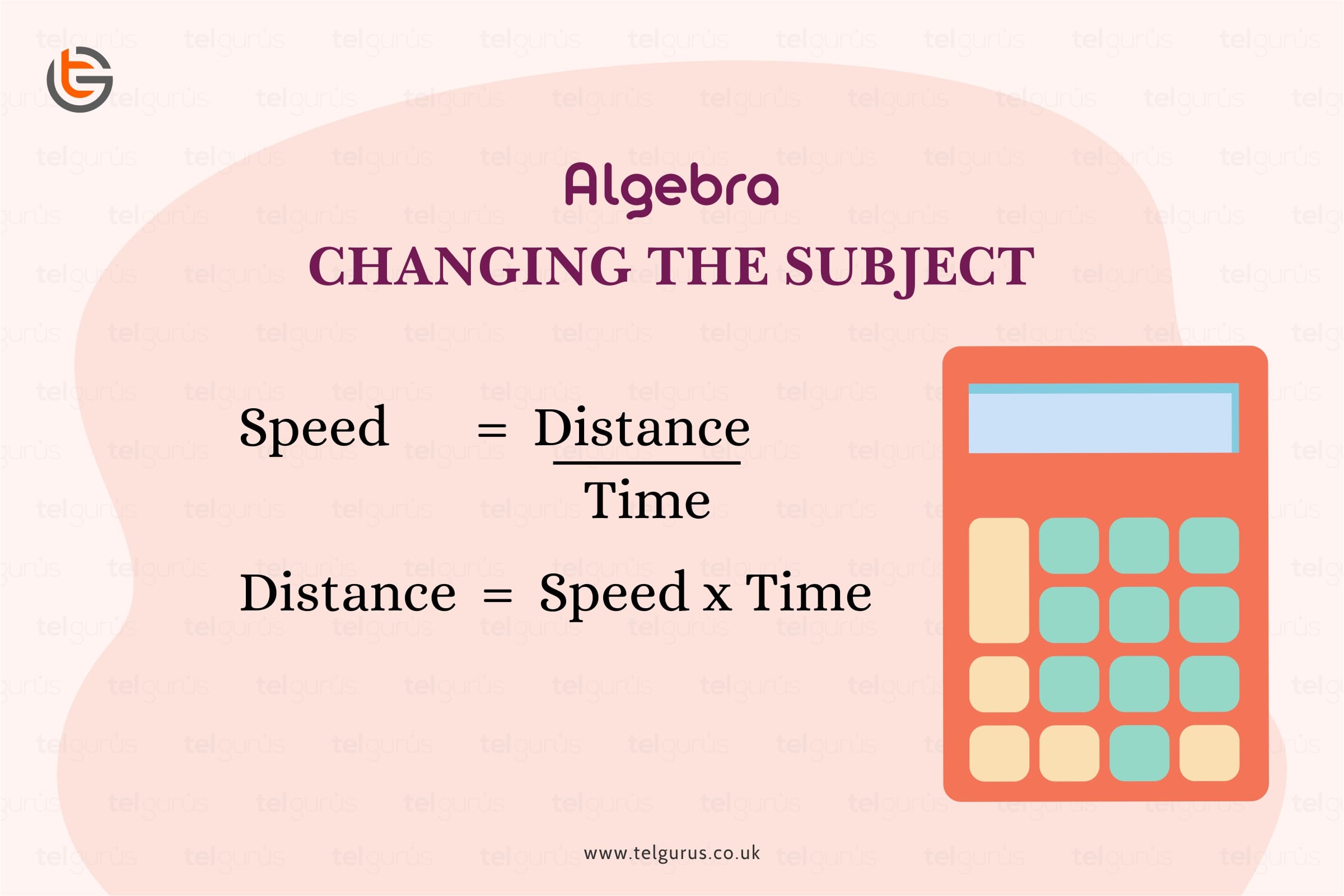
We know that, Speed = Distance/ Time or S = D/T 

In case we need to calculate Distance, we need to rearrange the formula to get Distance on left hand side and rest everything on right hand side. 

S = D / T 

On multiplying T (Time) on both the sides, we get 

S * T = D / T * T 

S * T = D 

Or, D= S * T 

Now, we get a single variable on the left hand side i.e. Distance.  

This way we can change the subject.   

• Involving squares/roots 

If A is area of a square with side S, we know that 

A = S2 

To calculate side, we will take square root on both the sides  

√A = √S2 

⇒ S = √A 

• Involving fractions  

If C stands for degree Celsius and F stands for Fahrenheit scale, we know the conversion rule i.e. 

(C – 0)/ 100  = (F -32)/ 180 …………………………….    (1) 

With this formula we can easily switch between the subjects and calculate what is desired.  

To convert Celsius scale to Fahrenheit scale, we will perform mathematical actions in such a way that F should be on one side and take the rest terms on the other side. 

Multiplying 180 on both sides on equation (1) 

C/100 * 180 = F-32 

Now, adding 32 on both sides and simplifying  

9C/5  + 32 = F 

So, the new temperature becomes F = 9C / 5  + 32. 

Similalr we can change the subject from F to C, by performing reverse operations which gives  

C = (F -32)* 5/9 

Let’s solve them using some practical examples 

Example1: Find the height of a cuboidal box, if its length is 10 cm, breadth is 45 cm and volume is 9000 cu cm.  

Solution: We know that V =  L *B *H 

But, here we need to find the value of h or height or make the main subject. 

In the above formula, we will divide both the sides with l and b 

Which gives us  

V/ (L *B) = H 

Substituting the values here, we get 

9000 / (45 * 10) = H 

H = 20 cm 

Height comes out to be 20 cm.  

Example2: Find the radius of a circle whose area is 314 cm2.  

Solution: We know the formula that 

\displaystyle A=\pi {{r}^{2}} where A is the area and r stands for radius. 

Here the main subject is A, we need to change the subject to r. 

For that we will divide both the sides with π  

Now taking square roots on both the sides, radius becomes  

r = √A/ π 

Now, Let now substitute the values given in the question to this derived.  

r = √(314/ 3.14) = √100 = 10 

The radius comes out be 10 cm.

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development 

Unleash the Power of visualization to break tough concepts

Wanna be the next Maths wizard? Discover the new way of learning concepts with real-life Visualization techniques and instant doubt resolutions.

Book A Demo Class

• 

  How is trigonometry used on non-right angled triangles?

• 

  Express 56 as the product of its prime factors?