How do you find and solve a composite function?

Book A Demo Class

To understand this, let us take an example from our daily life to explain this concept.

Suppose Harry went shopping for groceries at a supermarket. There he noticed that the discount on different items was dependent on the price range of that item.

He wondered how the price and discount were related.

In this case, the price is dependent on another function that is cost.

So, one function is dependent on another function. A function that depends on any other function is called a composite function. Composite functions are functions within the functions.

Suppose we are given two functions, with the help of these two functions we can create another function by composing one function into the other.

It is generally a function that is written inside another function.

Let us clear the concept with the help of an example.

Example

Let’s suppose that

$\displaystyle f(x)={{x}^{3}}$ and g ( x ) = x + 6 , defined for all real numbers .

We need to solve the equation f (g ( x )) = 125.

f(g(x)) is usually spoken as “f of g of x”.

Firstly, we must find the composite function f( g ( x )) in terms of x before we solve it.

In order to do this, we can break down the function in the following way:

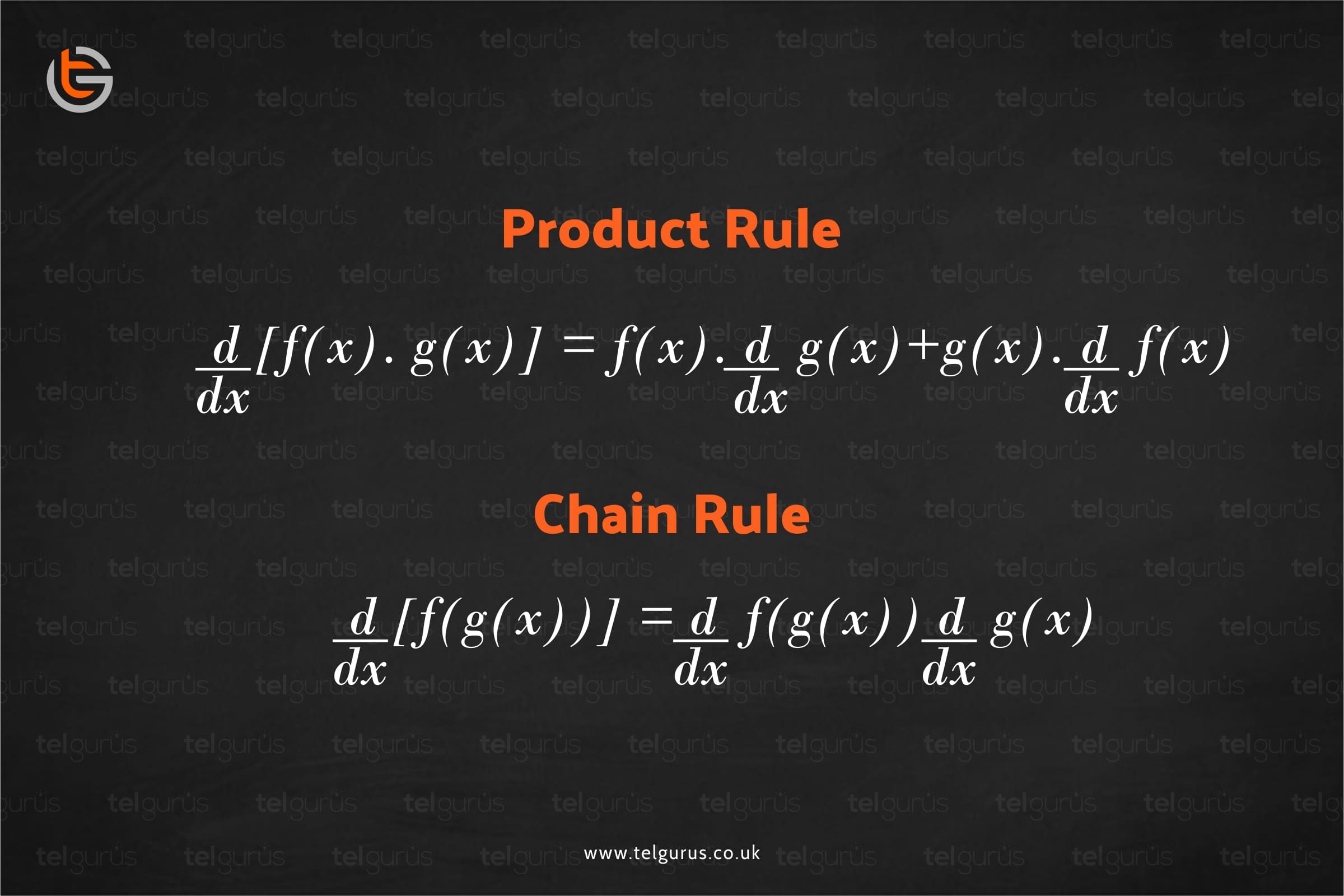
f (g ( x )) = f [ g ( x ) ]

= f ( x + 6 ) because value of g ( x ) is x + 6

Now we can see that we have broken down f g ( x ) into a function of g with in a function of f.

It’s time to replace ‘x’ in $\displaystyle f(x)={{x}^{3}}$ with x + 6

That is $\displaystyle f(g(x))={{(x+6)}^{3}}$

We can now use the above function to rearrange and solve for f g ( x ) = 125

Therefore $\displaystyle {{(x+6)}^{3}}=125$

x + 6 = ³√125

x + 6 = 5

x = 5 – 6

x = -1

Here, one thing is to be kept in mind is that the value of composite function f (g ( x )) is not the same as the value of the composite function g (f ( x )).

Both functions have different values. We can also denote these functions as ( f o g ) ( x ) where o is a small circle symbol.

Note that, we cannot replace o with (.) because dot will refer to the product of two functions.

Few properties of composite function

The function composition of one-to-one function is always one-to-one.

The function composition of two onto functions is always onto.
The inverse of the composition of two functions f and g is equal to the composition of the inverse of both the functions i.e. $\displaystyle {{(\text{ }f~o\text{ }g\text{ })}^{{-1}}}~\left( {\text{ }x\text{ }} \right)~\text{ }=~~\text{ }(\text{ }g{{~}^{{-1}}}~o~~\text{ }f{{~}^{{-1}}}~~)~~\left( {\text{ }x\text{ }} \right)$ .
Composite functions are not commutative, i.e. g o f (x) is not equal to f o g (x).
Composite functions are associative , i.e. taking three functions f, g and h: ( f o g ) o h = f o ( g o h )

Read More – Mathematics Questions

View More – Useful links for Your Child’s Development