What does expand mean in math?

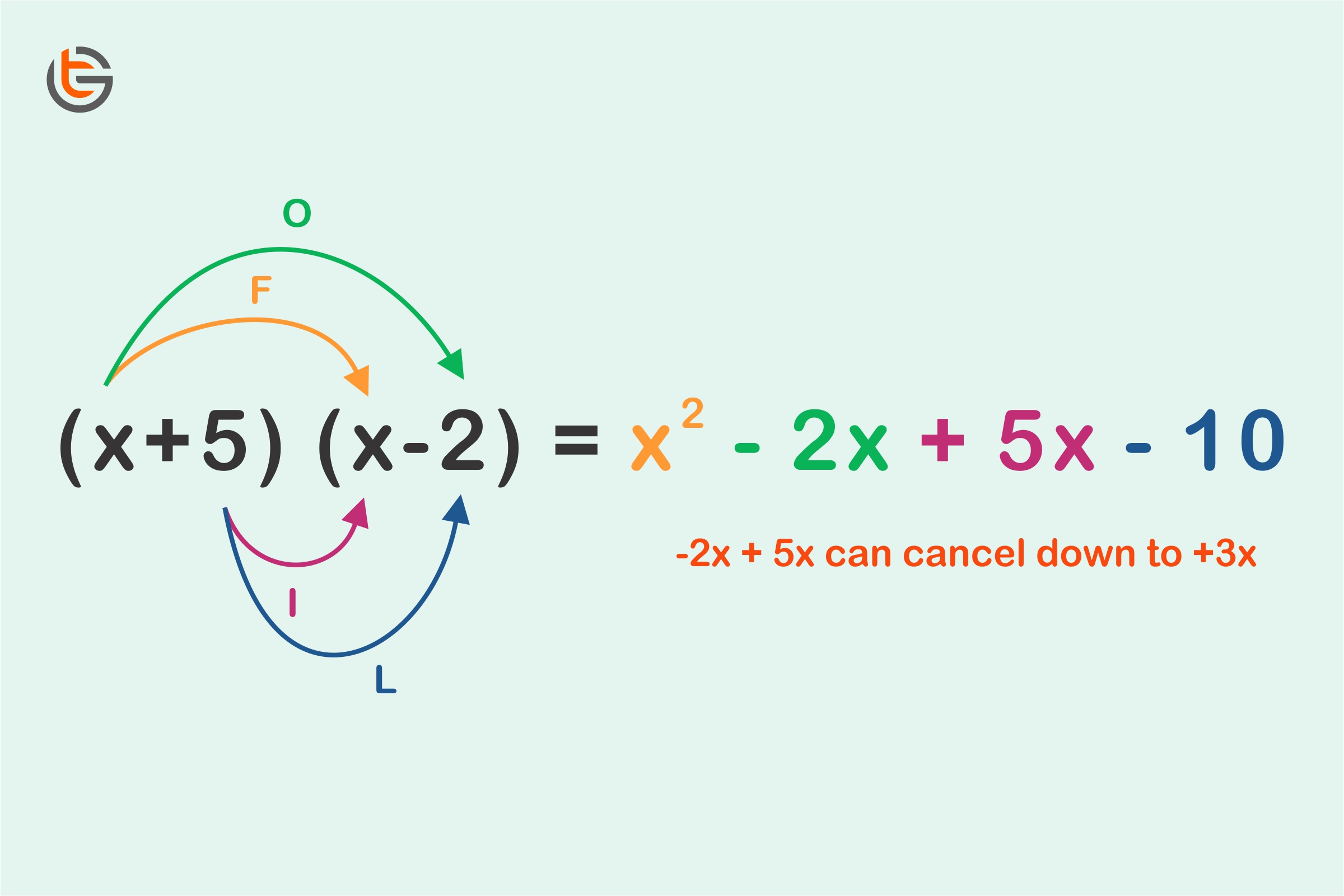
Expand in English means to make something larger or giving a fuller version of a thing.

Expand in math means opening the brackets ( ) or { } for arithmetic operations and simplifying the expression.

Tips to do that:

Whatever is inside the parentheses or brackets should be considered as a single term.
If we are performing multiplication every single term within the bracket would get multiplied with the expression written outside.

For example: on expanding $9(5+b)\text{ }\!\!\hat{\ }\!\!\text{ }$ we have to multiply 9 with both 5 and b. So, the expression becomes,

= 9 x 5 + 9 x b = 45 + 9b (remember the formula 3 x a = 3a where a is a variable)

Example 2: Expand ${10 (7y+9z)}$

Here, on opening the bracket we have to multiply 10 with both the terms inside.

We get = ${10 (7y+9z)}\,\text{=}\,\text{10 x 7y+10 x 9z}$

= 70y + 90z is the answer

Now, Let’s understand expanding algebraic terms and brackets with powers.

Power or indices indicate that how many times a number has been multiplied by itself.

For example: 2 x 2 x 2 can be written as ${{2}^{3}}$

Similarly, when a is multiplied by n times,

a x a x a… x a (n times) = ${{a}^{n}}$

Always remember, when we add multiply powers of same variables they get added.

Such as ${{r}^{4}}\,x\,{{r}^{5}}$ , Here the powers of r get added i.e. 4 + 5 = 9;

Or, ( r x r x r x r) x (r x r x r x r x r) = r x r x r x r x r x r x r x r x r or; $r9$

Example: Expand $5{{b}^{4}}\,(9{{b}^{2}}+8{{b}^{3}})$

For that we need to open the bracket and multiply $5{{b}^{4}}$ with $9{{b}^{2}}$ and $8{{b}^{3}}$ respectively.

On multiplying $5{{b}^{4}}$ with $9{{b}^{2}}$ we get

5{{b}^{4}}x\,9{{b}^{{2\,}}}\,=\,5x9x\,{{b}^{{4+2}}}\,=\,45{{b}^{6}}

And the On multiplying $5{{b}^{4}}$ with $8{{b}^{3}}$ we get

5{{b}^{4}}x\,8{{b}^{3}}\,=\,5x8\,{{b}^{{4+3}}}\,=\,40{{b}^{7}}

So, the answer becomes $45{{b}^{6}}\,+\,\,40{{b}^{7}}$

Things to remember

While simplifying and expanding any expression, you must follow the rules of BODMAS i.e. the order of mathematical operations which is brackets, indices, division, multiplication, addition and subtraction comes at last.
Multiplying two negative numbers would yield a positive number.
The powers or indices get added during multiplication and subtracted during division.
Additional and multiplication of variables can only be done when they have same powers. These are called like terms. There is no such thing for multiplication and division.

Problem1: Expand and simplify the expression: $-4({{b}^{{(2)}}}-8)+7{{b}^{{(2)}}}$

By following the rules of BODMAS, we will first open the brackets. So, the expression becomes