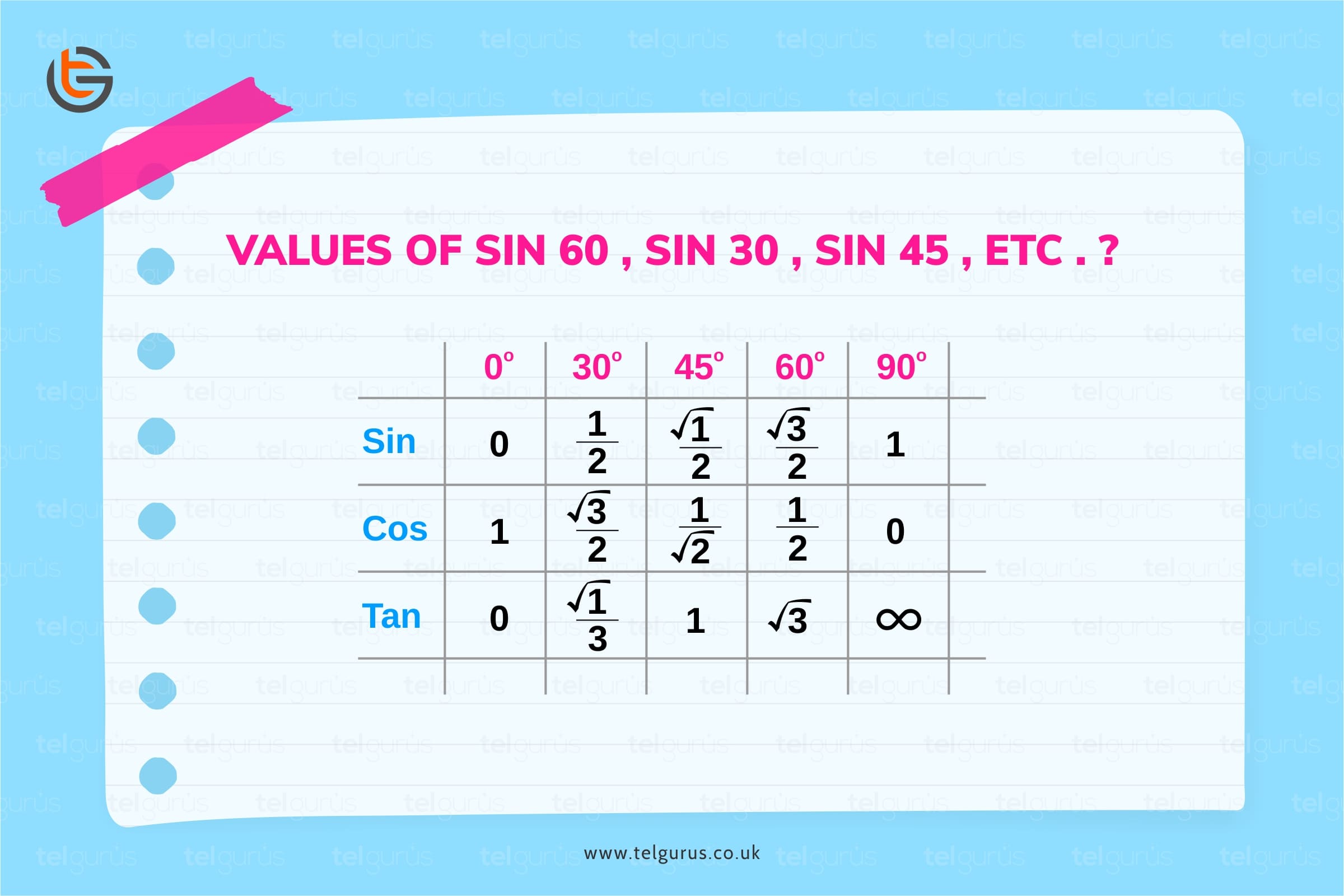
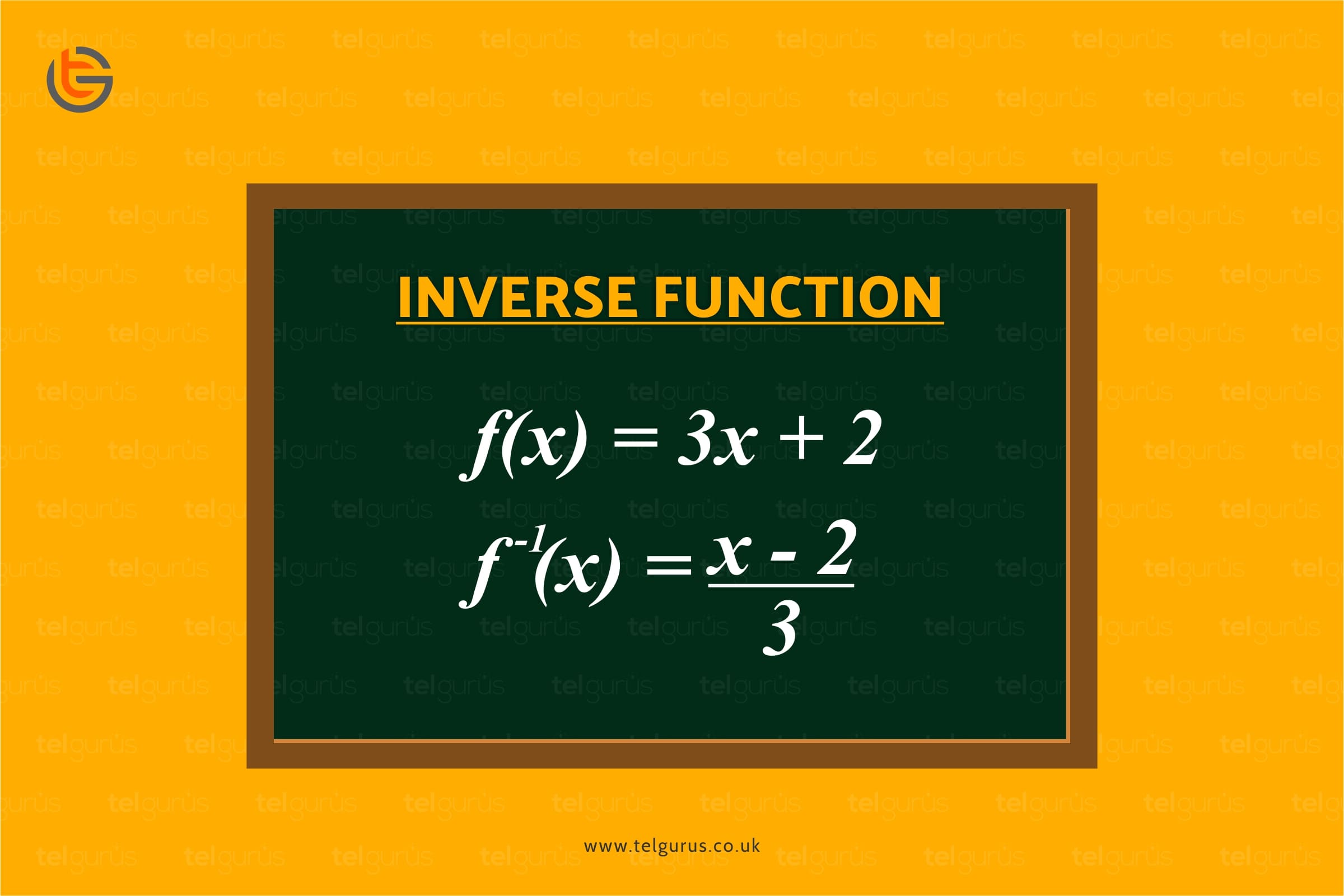
Enrich your knowledge with our informative blogs
List of common formulas that is commonly used for solving equations.

We know that an equation consists of an algebraic expression which is equated to zero.
The algebra formulas are just not used to solve these equations but form the foundation of various mathematical concepts.
Many topics such as quadratic equations, coordinate geometry, polynomials etc use these formulas for solution. These formulas help in simplifying the expressions and deducing the answers.
Before moving on to formulas, first understand what is Algebra and what are algebraic expressions.
What is Algebra?
Algebra is a more like a generalized arithmetic where letters are substituted for numbers.
The letters are known as variables and they don’t have a fixed value. The numbers are called constants as they have a fixed value.
Algebra is a wide branch of mathematics that covers vectors, matrices, complex numbers etc.
x, y, a and b are common letters that are used to represent the variables.
What is algebraic expression?
A combination of variables and constants which is connected with each other through various arithmetic operators such as +, -, x or ÷ is known as algebraic expression.
Basic Algebraic formulas to solve equations
-
- \displaystyle {{\left( {a+b} \right)}^{2}}~=~{{a}^{2}}~+~2ab~+~{{b}^{2}}
- \displaystyle {{\left( {a-b} \right)}^{2}}~=~{{a}^{2}}~-~2ab~+~{{b}^{2}}
- \displaystyle {{a}^{2}}-{{b}^{2}}=(a+b)(a-b)
- \displaystyle {{a}^{2}}~+~{{b}^{2}}~=~{{\left( {a~+~b} \right)}^{2}}-2ab
- \displaystyle {{\left( {a~+~b~+~c} \right)}^{2}}~=~{{a}^{2}}~+~{{b}^{2}}~+~{{c}^{2}}~+~2ab~+~2bc+2ca
- \displaystyle {{\left( {a~-~b~-~c} \right)}^{2}}~=~{{a}^{2}}~+~{{b}^{2}}~+~{{c}^{2}}~-2ab~+~2bc-2ca
- \displaystyle {{(a+b)}^{3}}={{a}^{3}}+3{{a}^{2}}b+3a{{b}^{2}}+{{b}^{3}}={{a}^{3}}+{{b}^{3}}+3ab(a-b)
- \displaystyle {{(a-b)}^{3}}={{a}^{3}}-3{{a}^{2}}b+3a{{b}^{2}}-{{b}^{3}}={{a}^{3}}-{{b}^{3}}-3ab(a-b)
- \displaystyle {{a}^{3}}-{{b}^{3}}=(a-b)({{a}^{2}}+ab+{{b}^{2}})
- \displaystyle {{a}^{3}}+{{b}^{3}}=(a+b)({{a}^{2}}-ab+{{b}^{2}})
- \displaystyle {{(a+b)}^{4}}={{a}^{4}}+4{{a}^{3}}b+6{{a}^{2}}{{b}^{2}}+4a{{b}^{3}}+{{b}^{4}}
- \displaystyle {{(a-b)}^{4}}={{a}^{4}}-4{{a}^{3}}b+6{{a}^{2}}{{b}^{2}}-4a{{b}^{3}}+{{b}^{4}}
- \displaystyle {{a}^{4}}-{{b}^{4}}=(a-b)(a+b)({{a}^{2}}+{{b}^{2}})
- \displaystyle {{a}^{{-m}}}=\frac{1}{{{{a}^{m}}}}
Formulas involving exponents
Let’s have a look at some very commonly used formulas for expressions having same base but different powers.
-
- \displaystyle {{a}^{m}}.{{a}^{n}}={{a}^{{m+n}}}
- \displaystyle \frac{{{{a}^{m}}}}{{{{a}^{n}}}}={{a}^{{m-n}}}
- \displaystyle {{({{a}^{m}})}^{n}}={{a}^{{mn}}}
- \displaystyle {{\left( {ab} \right)}^{n}}={{a}^{n}}.{{b}^{n}}
- \displaystyle {{a}^{0}}=1
- \displaystyle {{a}^{{-m}}}=\frac{1}{{{{a}^{m}}}}
Roots of quadratic equation
To find the roots of a quadratic equation of the form \displaystyle a{{x}^{2}}+~bx+c=0, you can use the following formula:
\displaystyle x=~\frac{{-b\pm \sqrt[{}]{{{{b}^{2}}-4ac}}}}{{2a}}Here, \displaystyle {{b}^{2}}-~4ac is known as discriminant or D.
D is helpful in finding the nature of roots of the equation.
- If D > 0, then the roots of the quadratic equation will have two real and distinct roots.
- If D = 0, then the two roots of the quadratic equation will be equal and real.
- If D < 0, then the quadratic equation will have two imaginary roots.
Let’s apply these using some examples:
Q1. Solve the expression \displaystyle {{25}^{2}}-~{{14}^{2}}
Solution:
For this question, no need to calculate the squares of the number. Just you need to apply the formula listed above.
Using \displaystyle {{a}^{2}}-~{{b}^{2}}=\left( {a-b} \right)\left( {a+b} \right)
We can write as:
\displaystyle {{25}^{2}}-~{{14}^{2}}=\left( {25-14} \right)\left( {25+14} \right)=11*39
=329
Q2. Solve the expression: \displaystyle {{5}^{2}}{{.5}^{3}}
Solution: By using the formula \displaystyle {{a}^{m}}.{{a}^{n}}={{a}^{{m+n}}}
\displaystyle {{5}^{2}}{{.5}^{3}}={{5}^{{2+3}}}={{5}^{5}}=3125
Read More – Mathematics Questions
View More – Useful links for Your Child’s Development

Unleash the Power of visualization to break tough concepts
Wanna be the next Maths wizard? Discover the new way of learning concepts with real-life Visualization techniques and instant doubt resolutions.
Categories
Recent Posts
- List of the qualities you should look for in your tutors?
- What is the most useful formulas in math?
- Describe the process of eating to defecation of food?
- Difference between the natural and artificial active response by the immunology system.
- Explain the different circle theorems
- How are nerve cells adapted to their function?